Prerequisite concepts
One can view $\frac{df}{dx}$ as approximately given by a fraction where $df$ is a small change in $f$ and $dx$ is a small change in $x$.
Technically, the derivative is a ratio of small changes in the limit that the change in the denominator goes to zero: $\frac{df}{dx}=\lim_{h \rightarrow 0} \frac{f(x+h)-f(x)}{h}$
One of Zandieh's process-object layers for derivatives is that derivatives are functions. This means that the value of a derivative depends on where in the domain of the function one is looking. The derivative of a function is itself a function, with the same domain as the original function. Both the (derivative) function and the value of the derivative at a point are commonly referred to as "the derivative."
Visualizing Divergence

The traditional discussion of divergence in vector calculus derives an algebraic expression in rectangular coordinates. We prefer to give a geometric derivation as flux per unit volume through an appropriately shaped box. In this activity, students learn how to predict the value of the divergence at any point by looking at the vector field near that point. The activity is an excellent one for fostering representational fluency, as students can be asked to engage with vector field maps on paper, plots in Mathematica, and symbolic expressions for vector fields. A nice extension is to have students consider vector fields that have different fundamental symmetries and to recognize that they can adjust the shape of the infinitesimal box to take advantage of such symmetry.
Representations used
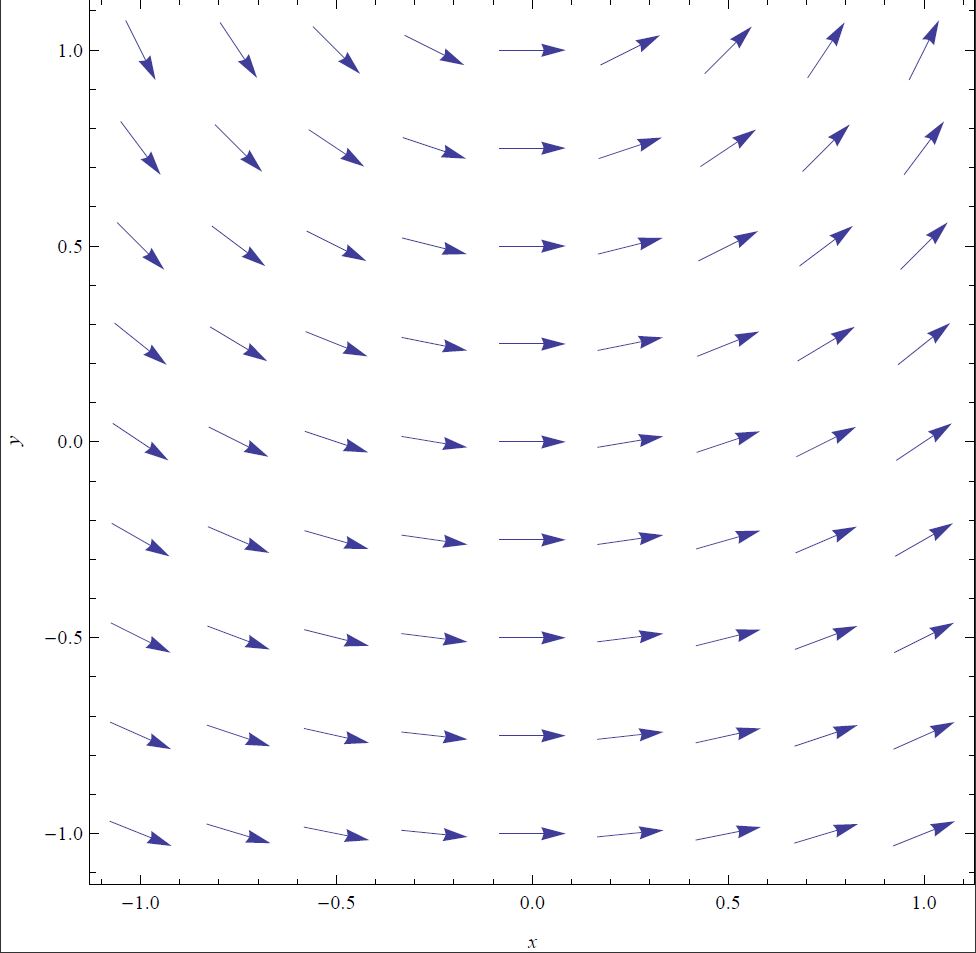
Concepts taught
If the total flux through a closed surface is not zero, then the divergence of the function at that point is non-zero.
The divergence of a function (unless taken at a single point) is itself a function
FIXME