Activity: Partial Derivative Machine Derivatives

Prerequisite concepts

Differential Calculus The derivative is a ratio of small changes

One can view $\frac{df}{dx}$ as approximately given by a fraction where $df$ is a small change in $f$ and $dx$ is a small change in $x$.

Variables can be held constant

When taking a derivative of a multivariable function, it is possible to consider a simpler situation in which some of the variables are considered to be constant.

Multivariable Calculus Derivatives can be found while holding one or more variables constant

Derivatives of multivariable functions can be found by holding one or more variables constant (subject to physical limitations).

The value of a partial derivative depends on the value(s) of what is held constant

FIXME: Is this really a statement about paths? I don't think we have path-related concepts.

Zapping with d

Differentials are small changes or differences

One typical notation used for derivatives is Leibniz notation ($e.g.,$ $df/dx$). Physicists often think of the $df$ and $dx$ as distinct quantities (``differentials'') that can be measured, calculated, and manipulated independent of one another. In particular, a differential can be thought of as the (small) change in a quantity when a small change is made to a physical system. A differential can also be thought of as the difference between the values of a quantity between two different (nearby) physical states. This line of thinking is also valuable for thinking about integration in physical situations.

Equations can be 'zapped with d' to relate differentials Individual differentials can be manipulated algebraically Total differentials are linear

Instructor guide inisowidth

Partial Derivative Machine Derivatives

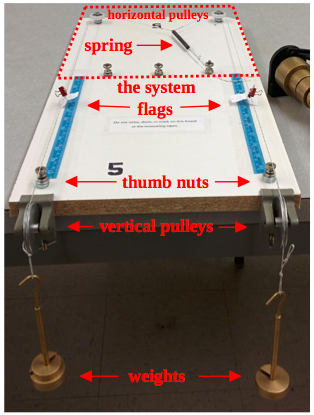
In this activity, students experimentally determine various derivatives using the partial derivate machine, a mechanical analogue for thermodynamic systems. Students explore the ratio, limit, and function aspects of multi-variable derivatives, with an emphasis on holding different variables constant. This activity is also an excellent exercise in representational fluency, as students must coordinate experiments and tables of data with (new) symbolic notations. $\textrm{Leibniz Notation}\ \frac{\partial f}{\partial x} \rightarrow \left(\frac{\partial f}{\partial x}\right)_y$

Representations used

PDM

$\frac{\partial f}{\partial x}$

$\left(\frac{\partial f}{\partial x}\right)_y$

$\begin{array}{c|c}x&y\\\hline3&0.2\\4&0.6\\5&0.9\end{array}$

Table

Concepts taught

There are experimental limits to how $small$ of a change can be measured

While it is relatively easy to imagine very, very small changes in physical values, there are often experimental limits on how small of a change can be measured. When designing and conducting experiments, there is a tension between these experimental limitations and normative representations of functions as smooth lines or

The derivative can be interpreted physically

While the constituents of a derivative (the $f$ and $x$ in $\frac{df}{dx}$) can have physical interpretations, the derivative itself can have a different physical interpretation. For example, $\frac{dx}{dt}$ is a velocity. FIXME: find a better example

Partial derivatives that do not have the same variable(s) held constant (at the same values?) are not the same derivative

FIXME

The coefficients in a differentials equation are partial derivatives

FIXME