Prerequisite concepts
One typical notation used for derivatives is Leibniz notation ($e.g.,$ $df/dx$). Physicists often think of the $df$ and $dx$ as distinct quantities (``differentials'') that can be measured, calculated, and manipulated independent of one another. In particular, a differential can be thought of as the (small) change in a quantity when a small change is made to a physical system. A differential can also be thought of as the difference between the values of a quantity between two different (nearby) physical states. This line of thinking is also valuable for thinking about integration in physical situations.
FIXME: What? One can do algebra with differentials and reinterpret them as partial derivatives. Can treat like a variable
Energy and Integrals

This integrated lab activity is designed to ask upper-division undergraduate students to measure the change in potential energy in an elastic system (PDM) between two different states. Students use the Partial Derivative Machine (PDM) to verify experimentally that the forces and dimensions of their system are state variables as well as measure the relationships between these quantities to compute the potential energy of their system. The whole class discussion focuses on the meaning of integration of discrete experimental data.
Representations used
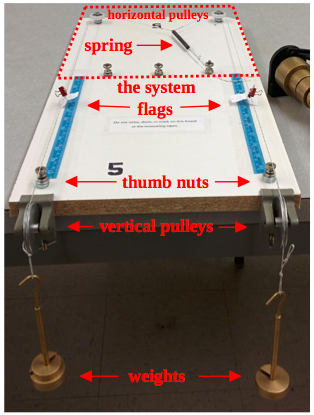
Concepts taught
$df$ can be viewed as a small chunk of $f$. When combined with 'The derivative is a ratio of small changes', it's easy to relate differentials to derivatives.
While it may be possible to conceptualize a particular derivative, such as $\left(\frac{\partial U}{\partial S}\right)_{V},$ Where $U$ is internal energy, $S$ is entropy, and $V$ is volume, that does not mean that the particular derivative can be directly measured. In the above example, the entropy $S$ is not measurable.