The colored bands indicate the resistance of any particular resistor.

If you know the resistance of individual resistors, you can calculate the equivalent resistance of a system of resistors, arranged in series and in parallel.

Consider 2 identical resistors in series.



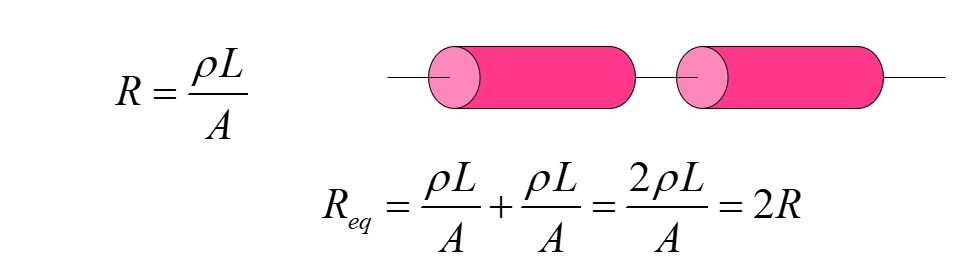
Assume we have zero resistance in the connecting wire. Since resistance is proportional to length, the equivalent resistor has double the length of either resistor. Adding them together just gives R_eq = 2R.



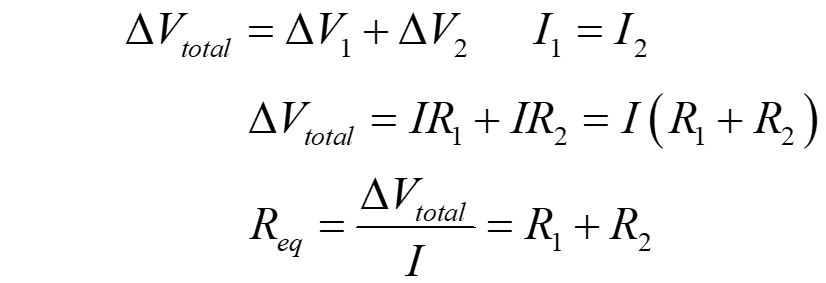
For any two resistors, we can use the facts that in series, the current through the resistors is the same current and the potential drops over the resistors add to equal the total potentials drop.

In series, two resistors just add: R_eq = R₁ + R₂. Now consider two identical resistors in parallel.



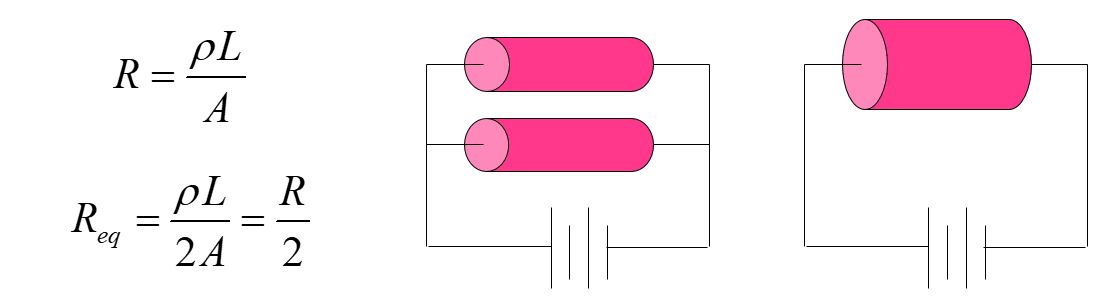
Now each resistor is connected separately to the battery. The equivalent resistor has doule the cross-sectional area of either resistor. Resistance is inversely proportional to the cross-sectional area, so the equivalent resistor has half the resistance of either individual resistor. Adding them together gives R_eq = R/2.



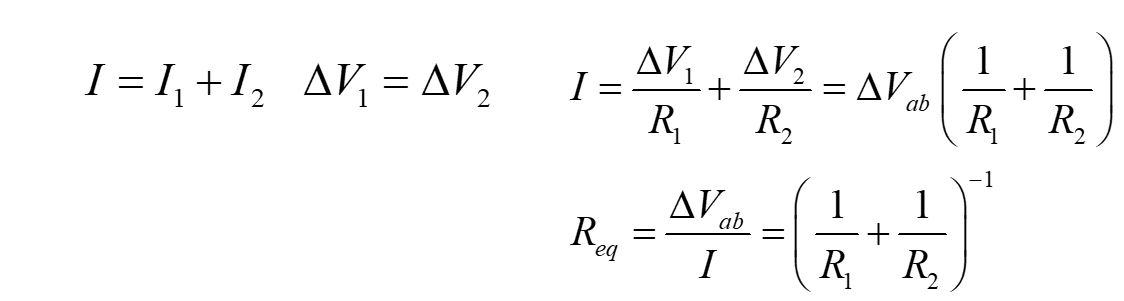
For any two resistors, we can use the facts that in parallel the potential drop across the resistors is the same and the current adds, to find the equivalent resistance.



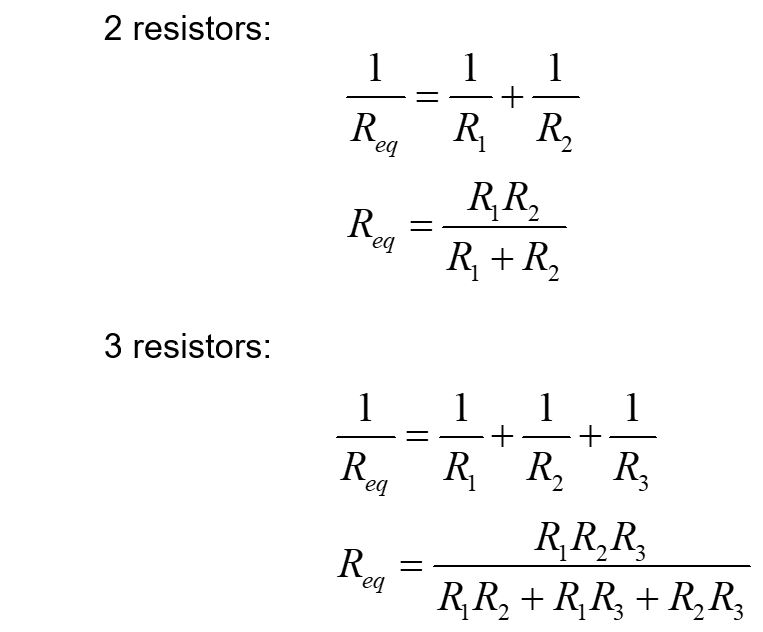
We use a little algebra to find a simple form for the equivalent resistance of two or more resisters in parallel.

Notice that the rules for adding resistors in series and in parallel are opposite the rules for adding capacitors.



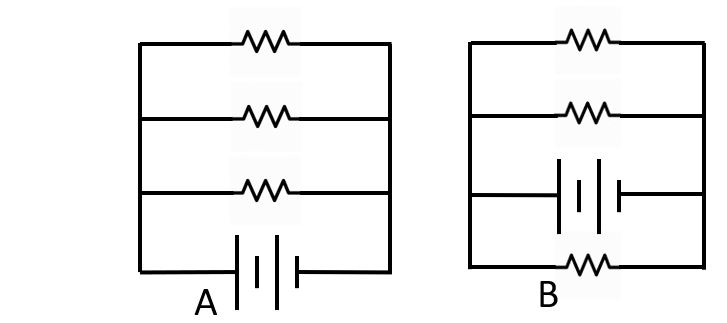
Resistors in parallel share a pathway to the battery. These two parallel resistor circuits are fundamentally identical, assuming identical resistors. It does not matter where the branch with the battery is placed, as long as the fundamental logic of the circuit is the same.

Sample questions

1. Which circuit is fundamentally different from the others?



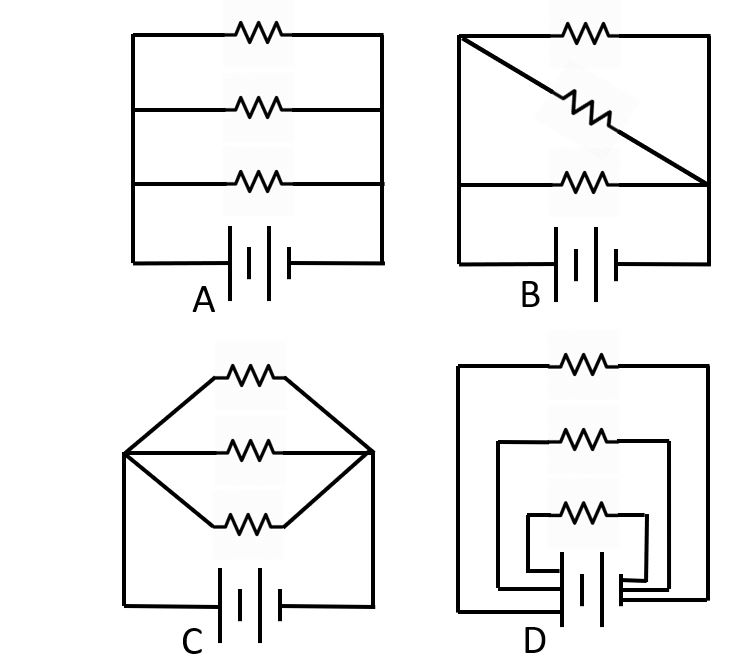
A. A

B. B

C. C

D. D

E. They are all the same



For the following questions, please consider identical light bulbs, and assume they can be treated as ohmic devices. Assume the batteries are ideal batteries.

2. Three identical bulbs are in series. When you unscrew Bulb B, what happens to the other two?



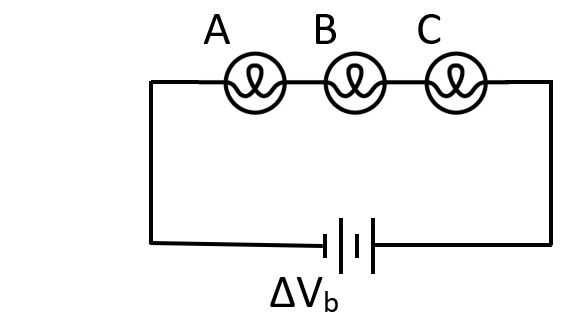
A. A stays the same, C goes out

B. A gets brighter, C gets dimmer

C. A and C are both unchanged

D. A and C both get brighter

E. A and C both go out



3. Three identical bulbs are in parallel. When you unscrew Bulb B, what happens to the other two?



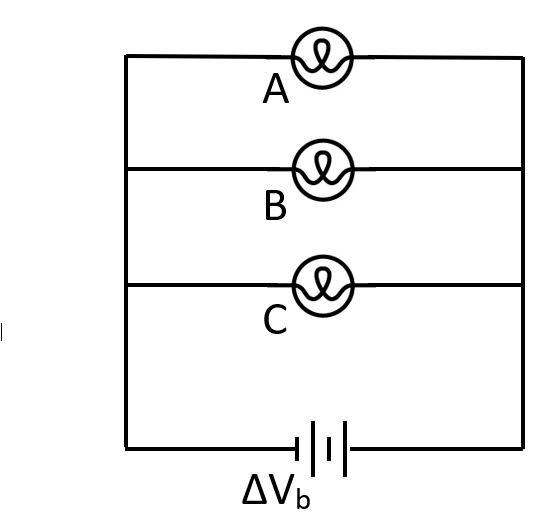
A. A stays the same, C goes out

B. A gets brighter, C gets dimmer

C. A and C are both unchanged

D. A and C both get brighter

E. A and C both go out



4. Rank the bulbs from brightest to dimmest.



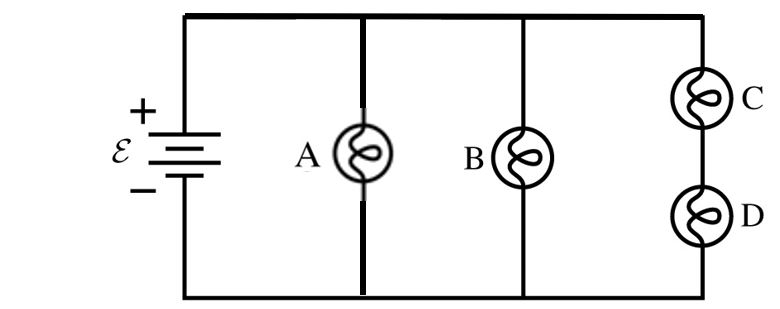
A. C = D > B = A

B. A = B > C = D

C. A = B = C = D

D. A > B > C = D

E. A > C > B > D



5. Rank the bulbs from brightest to dimmest.



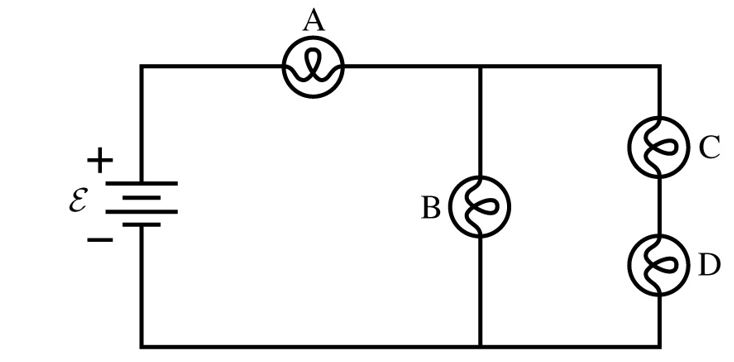
A. C = D > B > A

B. A > C = D > B

C. A = B = C = D

D. A > B > C = D

E. A > C > B > D



6. Rank the bulbs from brightest to dimmest.



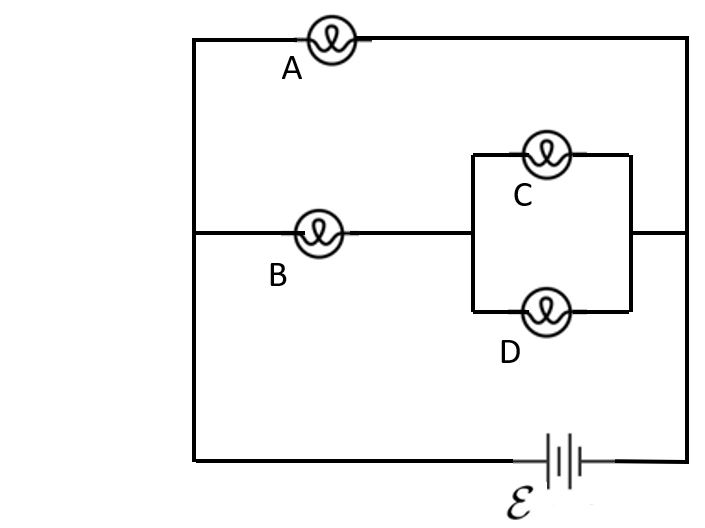
A. A > B > C > D

B. A = B > C = D

C. A > B > C = D

D. B > A > C = D

E. A = C = D > B



7. Rank the bulbs from brightest to dimmest.



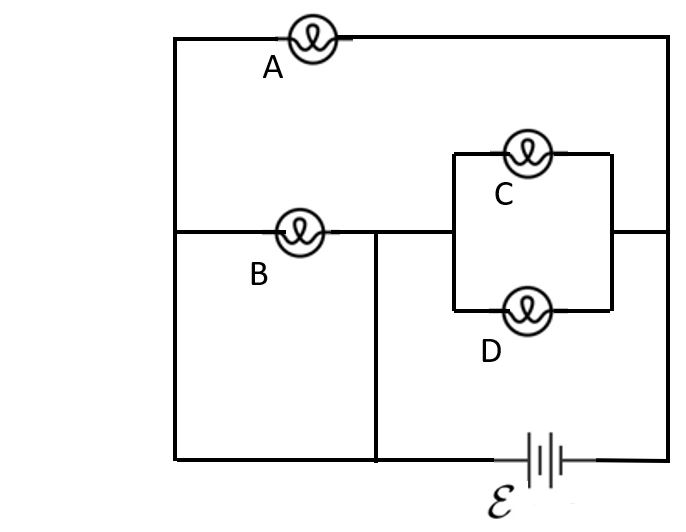
A. A > B > C > D

B. A = B > C = D

C. A > B > C = D

D. B > A > C = D

E. A = C = D > B



8. Find the currents in this circuit.



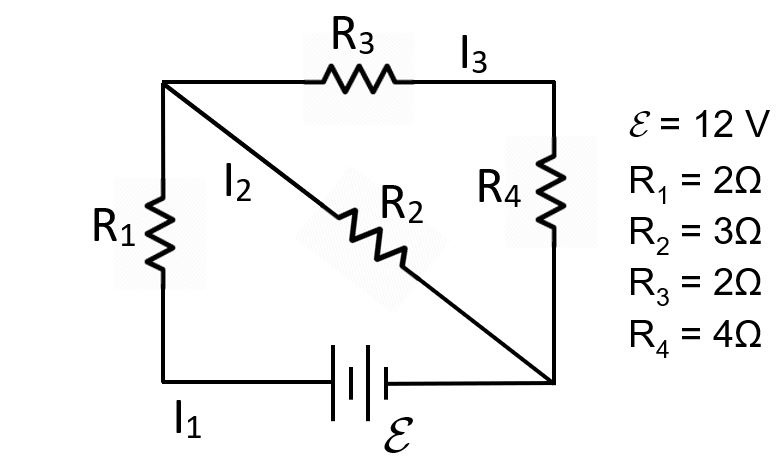
solution