The frequency $f$ of a beam of light is related to its wavelength $\lambda$ by the formula \begin{equation} f\lambda = \cc \end{equation} How do these quantities depend on the observer?

Consider an inertial observer moving to the right in the laboratory frame who is carrying a flashlight that is pointing to the left; see Figure 6.6. Then the moving observer is traveling along a path of the form $x'=x'_1=\hbox{const}$. Suppose the moving observer turns on the flashlight (at time $t'_1$) just long enough to emit 1 complete wavelength of light, and that this takes time $dt'$. Then the moving observer “sees” a wavelength \begin{equation} \lambda' = \cc \, dt' \end{equation}

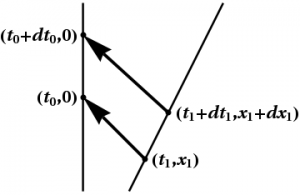Figure 6.6: The Doppler effect: An observer moving to the right emits a pulse of light to the left, which is later seen by a stationary observer. The wavelengths measured by the two observers differ, causing a Doppler shift in the frequency.

According to the lab, the flashlight was turned on at the event $(t_1,x_1)$, and turned off $dt_1$ seconds later, during which time the moving observer moved a distance $dx_1$ meters to the right. But when was the light received, at, say, $x=0$?

Let $(t_0,0)$ denote the first reception of light by a lab observer at $x=0$, and suppose this observer sees the light stay on for $dt_0$ seconds. Since light travels at the speed of light, we have the equations \begin{eqnarray} \cc (t_0 - t_1) &=& x_1 \\ \cc [(t_0+dt_0) - (t_1+dt_1)] &=& x_1 + dx_1 \end{eqnarray} from which it follows that \begin{equation} \cc (dt_0 - dt_1) = dx_1 \end{equation} so that \begin{eqnarray} \cc\, dt_0 &=& dx_1 + \cc\, dt_1 \\ &=& (\cish{dx'_1}+{\cc\, dt'_1}) + (\cish{\cc\, dt'_1}+{dx'_1}) \nonumber\\ &=& (\cish{}+{}) \, \cc\, dt'_1 \nonumber \end{eqnarray} since $dx'_1=0$. But the wavelength as seen in the lab is \begin{equation} \lambda = \cc \, dt_0 \end{equation} so that \begin{eqnarray} \frac{\lambda}{\lambda'} &=& \frac{dt_0}{dt'_1} = \cish{}+{} \nonumber\\ &=& \cosh\beta \, (1+\tanh\beta) = \gamma \, \left(1+\vc\right) = \sqrt{\frac{1+\vc}{1-\vc}} \label{freq} \end{eqnarray}

The frequencies transform inversely, that is \begin{equation} \frac{f'}{f} = \sqrt{\frac{1+\vc}{1-\vc}} \end{equation}