This page has the following sections:
Why Make a Change of Variables?
Consider the following double integral
![]()
where R(xy) is the region shown in the plot below. (A,B,C, and D are labels for the 4 sides.)

The region is neither vertically simple nor horizontally simple. The region can be described mathematically as the union of two vertically simple regions. We have
![]()
![]()
We must write the double integral as sum of two iterated integrals, one each for the left and right halves of R. We have
![]()
In some cases it is advantageous to make a change of variables so that the double integral may be expressed in terms of a single iterated integral.
Example of a Change of Variables
There are no hard and fast rules for making change of variables for multiple integrals. We proceed with the above example. It is appropriate to introduce the variables:
![]()
The INVERSE transform is
![]()
This is an example of a linear transformation. That means lines in the xy plane are transformed into lines in the uv plane. This particular change of variables converts the diamond shaped region R(xy) in the xy plane into a square R(uv) in the uv plane. Why? Well, the line x+2y=2 is transformed into the line u=2, and the line x+2y=-2 is transformed into the line u=-2. The two other lines are transformed into the lines v=2 and v=-2.
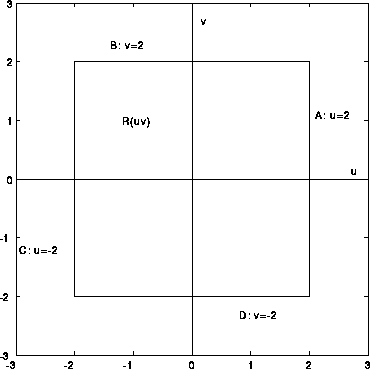
The new integrand is:
![]()
Before we can write down the new integral, we must introduce the Jacobian, which relates infinitesimal areas in the xy plane to infinitesimal areas in the uv plane.
The Jacobian is a function relating infinitesimal areas in the xy plane to infinitesimal areas in the uv plane. Why is this function needed? Notice that the area of R(uv) in the uv plane is 16 and the area of R in the R(xy) plane is 4. It can be shown that
![]()
(Check for yourself.)
There is a Jacobian in one dimensional calculus. Suppose that a change of variables x=g(u) is made converting an integral on the x-axis to an integral on the u axis. Suppose that u=G(x) is the inverse tranformation. Then:
![]()
The Jacobian is g'(u). This function relates infinitesimal intervals on the x axis to infinitesimal intervals on the u axis. If x=g(u), then dx=g'(u)du.
A Jacobian is required for integrals in more than one variable. Suppose that
![]()
Let us see what happens to a small infinitesimal box in the uv plane.
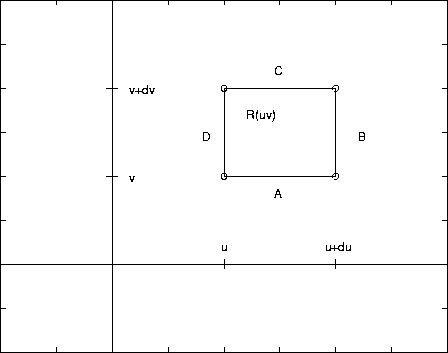
Since the side-lengths are infinitesimal, each side of the box in the uv plane is transformed into a straight line in the xy plane. The result is that the box in the uv plane is transformed into a parallelogram in the xy plane.
Suppose that the point (u,v) is transformed into the point (x=f(u,v),y=g(u,v)). The point (u+du,v) is transformed into the point
![]()
![]()
Here we have used Taylor series to expand these expressions. Similarly, the point (u,v+dv) is transformed into the point
![]()
This is shown in the figure below.
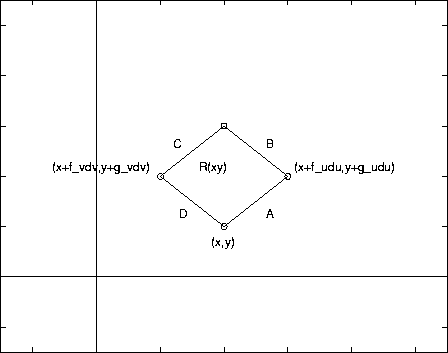
If we let S denote the vector from (x,y) to (x+f_udu,y+g_udu), S=<f_udu,g_udu,0>, and T denote the vector from (x,y) to (x+f_vdv,y+g_vdv), T=<f_vdv,g_vdv,0> then the area of R in the xy plane is |SxT|, where x denotes the cross product. It can be shown that
![]()
The quantity dudv is the area of the box R(uv). Hence,
![]()
The quantity
![]()
is called the Jacobian, which relates areas in the uv and xy planes. An equivalent formula for the Jacobian is
![]()
Here det means the determinant.
The correct formula for a change of variables in double integration is
![]()
In three dimensions, if x=f(u,v,w), y=g(u,v,w), and z=h(u,v,w), then the triple integral
![]()
is given by
![]()
where R(xyz) is the region of integration in xyz space, R(uvw) is the corresponding region of integration in uvw space, and the Jacobian is given by
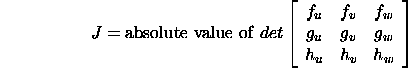
For the example considered above, we have
![]()
and
![]()
The Jacobian is J=1/4, and it follows that
![]()
where D is the square -2<=u<=2, -2<=v<=2. Hence, we have
![]()
It can be shown that the value of the integral is 48.
See the related page for computations of the Jacobian for the transformations to polar and spherical coordinates.
Copyright © 1996 Department of Mathematics, Oregon State University
If you have questions or comments, don't hestitate to contact us.