You are here: start » courses » lecture » cflec » cflecclassicallim
The Classical Limit Lecture ( minutes)
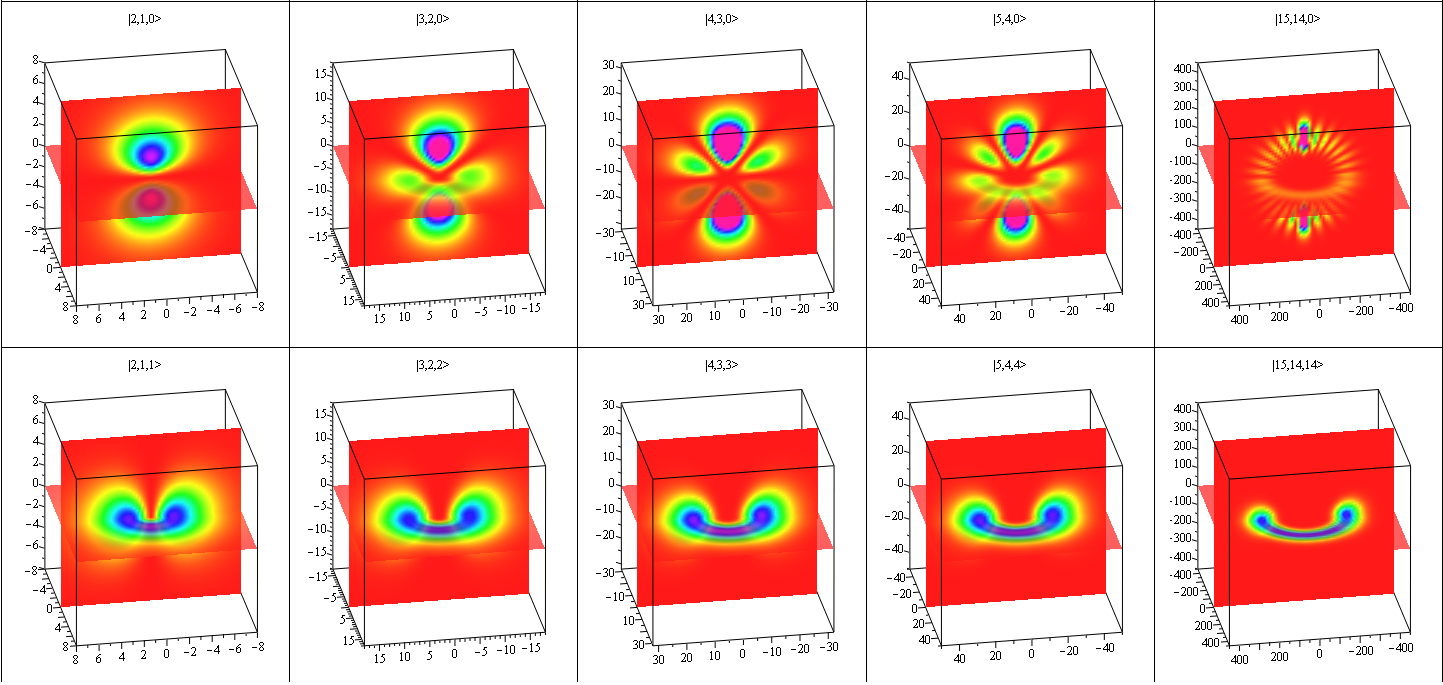
- Discussion of what happens to the $Y_{l,m}$'s as $l$ and $m$ get very large? In particular looking at the cases for which $m=0$ and $m=l$.
- Small whiteboard question: Find the expectation value of r in the 1S state. After students work on/struggle with this for 2-3 minutes, it is often helpful to discuss with them how one can do calculations when the operator used in the calculation does not share eigenvalues with the hamiltonian.
- The calculation of $\langle r \rangle$ leads to a discussion of the limiting case for $\langle r \rangle$ when n is very large. $\langle r \rangle = a_0 n^2$
- How localized particles, as opposed to the spread out wave