Understanding how a graph of effective potential is connected to actual motion.

Estimated Time: 15 minutes

A student is invited to “act out” motion corresponding to a plot of effective potential vs. distance. The student plays the role of the “Earth” while the instructor plays the “Sun”.

• An introduction to graphs of energy vs. distance.
• An introduction to angular momentum and its conservation in orbital motion.

• An energy graph (energy vs. distance) of the effective potential of a generic planetary orbit drawn on the blackboard.

We typically begin this activity with a lecture about the classical equations of orbital motion, specifically a derivation that reformulates 2-D equations of orbital motion to 1-D by using the effective potential.

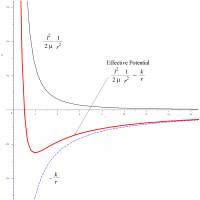
Just before initiating the activity, the following plot is made on the board as a reference for the student. It may be useful to contrast this plot with one for the harmonic oscillator since that case is the most familiar potential energy plot.

This is an 8 minute video clip of the activity cfveffkin.wmv

• It is important to select the student “volunteer” carefully. It seems to work best when the volunteer is confident enough to be involved in a discussion in front of the class (without being overly embarrassed about making mistakes), but also will not immediately come to a correct answer. Avoid students with a strong interest in astronomy.
• We often find that the student volunteer will initially act out the orbital motion based on the common knowledge that planets make elliptical orbits. The volunteer should be directed to explain how this motion is related to the graph. (You can ask, “Is that what the graph says?”).
• Almost all students interpret the graph to be back-and-forth oscillatory motion - and laughter often accompanies this clearly inaccurate orbit.
• The student volunteer should be specific about how the graph indicates how close/far he/she gets to the “sun”.
• The student volunteer should be asked to identify where the “sun” is located on the energy graph. This helps the student to understand what it means to fully articulate the meaning of the graph.

No separate wrap-up discussion is necessary, but the take home message should be that the plot of the effective potential has buried in it information about the angular momentum, and that the corresponding energy graph only tells you about the radial motion.

It is valuable to discuss the specific parts of the conservation of energy equation $$E = {{1}\over{2}} \mu \dot{r}^2 + {{1}\over{2}} {l^2 \over \mu r^2} - {k \over r}$$ explicity identifying the effective potential ${{1}\over{2}} {l^2 \over \mu r^2} - {k \over r}$, the radial kinetic energy ${{1}\over{2}} \mu \dot{r}^2$, and the $\phi$ component of the kinetic energy ${{1}\over{2}} {l^2 \over \mu r^2}$.

This activity works particularly well when sequenced with other activities.