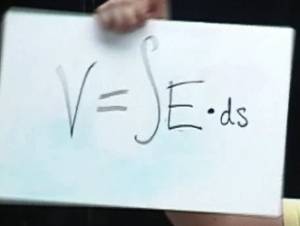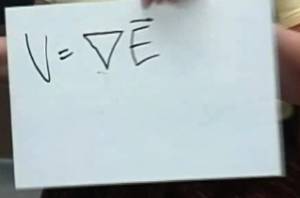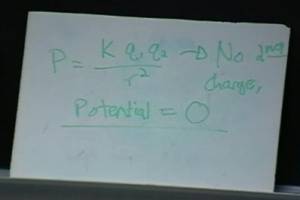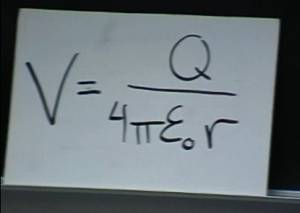This narrative provides an example of a discussion early in the school year when the instructor used a small white board question to engage students in actively thinking about pertinent issues. This session occurred on Thursday, September 27, 2007.

When students enter this classroom, each picks up a small white board (about 30×40 cm), marker, and cloth to use as an eraser. When the instructor asks a small white board question, the students write their answers on these small white boards. Sometimes they just hold up their boards so that the instructor can see the array of responses and make a quick assessment of the level of knowledge present in the group.

Sometimes, however, the instructor moves around the room as the students are writing on their small white boards. As the students finish, the instructor picks up examples representing various issues needing attention and places these, with writing facing away from the students, in a particular order along the chalk tray of the blackboard at the front of the room. The responses written on each of these selected small white boards then form the basis for the subsequent discussion.

During a discussion, the instructor also sometimes asks individual students to write on their small white boards as a way to help them communicate their ideas. Occasionally a student will spontaneously write on a small white board to clarify a question the student wants to ask.

Examples of these uses of small white boards are provided here. This discussion occurred during the first week of the academic year, when the instructor was still establishing how students should think and behave now that they had reached upper level courses as physics majors. There were 21 students in this class, including two women, both of whom contributed to the discussion interpreted here.

The first course in the Physics Paradigms program, Physics 320, emphasizes the use of symmetry and idealizations in problem-solving. The physics contexts include Gauss’s and Ampere’s Laws in orthonormal coordinates. The course meets for one hour on Mondays, Wednesdays, and Fridays and for two hours on Tuesdays and Thursdays. During the course described here, the instructor was Corinne Manogue. She has described the first three days of the course as follows:

Day 1 began with a discussion about forces and idealizations in the context of the equations of motion for a pendulum and why you might want to use a power series in that description. The idealization is that the angle by which the pendulum is displaced is small. After a discussion based on the students responses to a small white board question about the integral of sine theta square, Corinne presented a short lecture about power series, She ended the class with a discussion about working in groups and why it might be useful to do so.

Day 2 started with a small group activity about calculating the power series coefficients. Next the students learned about how to use Maple, a computer algebra system with nice graphics capabilities. Then small groups used Maple to learn about how to visualize power series approximations.

During Day 3, Corinne talked about why one cares about power series and introduced the idea that one could use them to solve integrals that one can not solve otherwise. There was a small white board question that led to a discussion about dot products and about the representation of vectors and the idea that you use the dot product of a vector with itself to find its length. . She was setting up a classroom norm about having a discussion based on small white board questions.

The narrative presented here focused upon developing an algebraic expression for the electrostatic potential due to a point charge. This small white board discussion occurred during Day 4. To help the students visualize the issues to be articulated, the instructor, Corinne Manogue, had assembled the following on a supply table at the side of the room: a hand-sized ball to represent a point charge, a voltmeter with a probe, three dowel rods connected at right angles and labeled x, y, z, to represent a rectangular coordinate system, a small white ball to represent a test charge, and a basketball to represent a second large charge. She used these props to model the physics to be discussed such as the concept of electrostatic potential and the various distances involved in its mathematical representation.

In reflecting upon this video, Corinne noted that in an earlier year she had asked students to write the formula for electrostatic potential as a five-minute warm-up at the beginning of the first day of class. She and the students had spent the whole hour on that question, however, because the students had not remembered the formula and in trying to come up with it confused the concepts of force, potential, energy, and potential energy. They also had not known how to talk about the dependence of the electrostatic potential on distance from a point charge.

Corinne no longer assumed that the students remembered what they had ‘learned’ previously and designed the instruction summarized above for Days 1-3. This had meant delaying asking for the formula for electrostatic potential for a point charge until Day 4. By asking for the formula as a small white board question, Corinne could tap the knowledge of all the individuals in the class. Because she expected the students’ answers to confuse force, potential, potential energy, and electric field, she intended this discussion to get the students to reason about the differences among these concepts as well as to develop the expression, $V = \frac{kq}{4πε_0 r}$, for the electrostatic potential due to a point charge.

During the early part of Day 4, Corinne had engaged the students in thinking about how to refer to two particular points in space and the distance between them. To create a memorable context, she had embedded this topic in a scenario based on a popular TV show, Star Trek. Although now a classic playing in reruns, the show was well known to most of these students. She invited two students who said they were familiar with the show to play the roles of Mr. Spock and Captain Kirk. She selected two students sitting on opposite sides of the classroom because in the scenario she was creating Spock and Kirk had become separated while exploring a planet. Standing on a table to represent being in the space ship, Corinne was playing the role of Scotty. Scotty needed to know where Spock and Kirk were on the planet in order to ‘beam’ Spock to help Kirk, who was battling aliens.

Corinne intended this scenario to emphasize the importance of specifying an origin, identifying vectors from the origin to two particular points in space, using these position vectors to formulate a vector representing the distance from one point to the other, and taking that vector’s dot product to calculate the distance between the two points. The students would need to be able to do this in developing an expression for the electrostatic potential at a particular point in space due to a point charge located somewhere other than a designated origin.

Teaching such mathematics through role-playing was more than simply a way to enliven what might otherwise have been a tedious presentation of procedures for using vectors. The Star Trek scenario, particularly with an instructor standing on a table, signaled that this class was not going to be like any that the students had experienced before. The scenario created a humorous open environment where students could contribute to the thinking without experiencing much anxiety. This established a friendly atmosphere for launching the discussion that followed, where the students would need to respond to a question on their small white boards, each publically revealing the level of knowledge known or not known. This small white board discussion was a way of talking with the students about what the formula for the electrostatic potential should look like and comparing and contrasting the students' answers.

Corinne designed this discussion to develop several ways of thinking about how one learns physics. Although introductory physics is often perceived as being about templates for problem solving, this class would be about reasoning and questioning was encouraged. She wanted the students to recognize that most people in the class do not remember things so the students should not feel alone in the fact that they cannot remember a formula from introductory physics, that even if they cannot remember the formula, they may remember parts of it, and that they have resources for reasoning about a formula and deciding whether or not they believe their memory. She intentionally models for them making those kinds of judgments and talks with them explicitly about making those kinds of judgments. She asks the open-ended small white board question so that lots of people write different kinds of things. She tries to validate the answers of people who do not necessarily write down what she intends and talks with them about how those answers might be relevant later. She is intending to send the signal that no knowledge is wasted, even if it is not needed right at the moment

The interpretative narrative below is based upon a transcript of the small white board discussion and upon reflections recorded by the instructor, Corinne Manogue, and her colleagues while viewing a video of this session. Elizabeth Gire was a postdoctoral fellow who had taught, or co-taught with Corinne, several Physics Paradigm courses; she had been present during this class. Kerry Browne had conducted his dissertation research in the context of earlier Physics Paradigm courses; he watched the video with Corinne briefly during a visit. The narrative was drafted by Emily van Zee, a science teacher educator who drew upon her own teaching experiences in laboratory-centered physics courses for prospective teachers and her research in the tradition of ethnography of communication (Hymes, 1972; Philipsen & Coutu, 2004; van Zee & Minstrell, 1997a,b). Ethnographers of communication examine cultural practices by interpreting what is said, where, when, by whom, for what purpose, in what way, and in what context. This interpretative narrative presents an example of an instructor initiating students into the culture of physics, specifically into the verbal and mathematical language that physicists use in describing electrostatic potentials.

Having reviewed the mathematics of working with vectors in an intriguing fictional context, Corinne began discussion of electrostatic potentials with a transition between the Star Trek scenario and the new topic:

Corinne: We didn't get Mr. Spock to Captain Kirk in time and the aliens have blown up everything in the universe. The only thing which is left is this single charge.

Corinne held up a hand-sized ball that represented the single charge.

Corinne: This is the only thing in the universe. Are we idealizing the universe? Yes. We have one charge in the whole entire universe.

Then Corinne held up a voltmeter with a long wire probe attached.

Corinne: Here we are idealizing a volt meter. All right?

So you must not tell my experimentalist friends what we're going to do because we're going to pretend that the voltmeter actually measures potentials and it doesn't. It measures currents. All right, but we're going to ignore that for the moment; It's called a voltmeter and we're pretending that it measures what we think.

A student interjected a question:

Student: What are the units of potential?

In reflecting upon this student question, Liz wondered why the student asked it. Corinne responded that she hoped he was sense-making, that she had just raised the question of what a voltmeter measures and though she had said it measures potential, the question remained, what is potential? This was a question that she had had herself as a student. She had not realized that a potential, as in what a potential is with respect to a point charge, had anything to do with what a voltmeter reads in a circuit, so it was possible that she had set up that same conflict in this student’s mind.

Corinne acknowledged the student’s question but deflected answering it back to the student.

Corinne: What are the units of potential? That's part of your homework for tomorrow. Look it up. Excellent question. That's why I ask it of you. (laughs) Ok. So what are the units of potential is something that you'll be discovering for tomorrow.

Then she moved on with her agenda to introduce electrostatic potentials by posing a small white board question while holding up the probe connected to the voltmeter in one hand and the ball representing the point charge in the other.

Corinne: But I have my voltmeter and I want you on your little white boards to write down as I move this probe around and I'm measuring the potential, I want you to write a formula for what I'll get.

We're actually doing some physics instead of mathematics just for the fun of it.

While watching this segment of the video, Liz wondered how careful Corinne had been with the particular way she had worded the question. Corinne responded that she had been very careful and noted that she had taken a lot of time to set the question up with manipulatives so that she was modeling the geometry of the situation with actual objects (a ball, a voltmeter, and a wire with probe attached to the voltmeter). She also had taken the time to acknowledge the idealizations she was making, partly because the course is explicitly about idealizations but also partly because she wanted students to recognize when idealizations were being made. So she made sure they understood that there was nothing else in the idealized universe besides that charge. She also made sure they understood that she was not trying to understand how a voltmeter really works.

Corinne was asking the small white board question to get students to go from a physical representation to an algebraic formula. She had asked explicitly for a formula to signal that she was not looking for different representations for one concept. Here she was looking for a correct answer (which was not always the case with small white board questions). She was using physical objects, holding up the stationary ball while moving the probe around to different positions, and asking them to write down a formula for what the probe would be measuring.

Commenting that Corinne had not read the question nor written it on the board, Liz asked how scripted the question had been. Corinne noted that this question had been planned and refined over many years. She had not scripted the introduction, although there were points she had planned to make, but the actual question, “I want you to write down a formula,” matched the script that was in her head. She noted that she does not write on the board as much as other people, so she expects the students to be constantly alert; she also commented that she thinks speaking small white board questions, rather than writing them on the board, allows the class to be more free flowing.

In spite of the care that Corinne had put into designing and stating the question, a student asked for clarification:

Student: What did you want us to write?

Still holding up the ball in one hand and the probe to the voltmeter in the other hand, Corinne restated the question:

Corinne: This charge is making an electrostatic potential everywhere in space, which I can measure with the probe of a voltmeter, if it really were a voltmeter. I want you to write a formula for what I'll get as I move this probe around.

She added:

Corinne: And write big enough that I can harass you by looking at your answers.

This was a form of coaching early in the term, to shape the students’ writing on the small white boards so that responses could be read by students and instructor from across the room. Corinne also was using humor to signal that she would be reviewing and actively discussing the students’ responses.

A student posed a more specific question:

Student: Do you want the potential or the electric field?

Corinne: Potential

Student: In terms of the electric field?

Corinne: Have I mentioned electric field yet?

Student: You mentioned charge

Corinne: I mentioned charge so write it in terms of charge.

Student: (?)

Corinne: I don't care because I'm going to hold up a lot of answers so you can all tell me something.

This was another statement that was a form of coaching these students into the culture of a Physics Paradigms classroom. Corinne was signaling explicitly what was about to happen, that the responses the students were writing on their small white boards would form the basis for the upcoming discussion, one in which all would be expected to participate.

Liz noticed that Corinne was asking for a formula but the voltmeter does not display a formula, it displays a value of the potential. Yet the students seemed to know exactly what Corinne meant, they did not seem to be having any trouble with this. Liz also wondered why the student had asked about the electric field, given how clearly Corinne had asked for the potential. Corinne interpreted that as evidence of his cognition, that he was realizing himself that these are two different things. Liz suggested that the student questions seemed to be oriented around what kinds of things could be included in the formula, that they might be asking what are the knowns and unknown. Corinne agreed that this is a norm, standard in physics lower division problem solving, to realize that the knowns are the things that are in the statement of the problem.

As the students were responding on their small white boards, Corinne walked around the room to see what they writing. As she picked up one of the small white boards, a student expressed uncertainty but Corinne affirmed she wanted to use the response “even if you do want to rethink it.” She placed the white boards she had collected on the chalk tray of the blackboard in front of the room, with the writing toward the blackboard so that the answers could not be seen. As she continued to walk around the room while collecting more small white boards, she stated explicitly what she was doing and why, again a form of coaching her new students into the culture that she was establishing in this classroom.

Corinne: All right. One of the questions that came up. I'm collecting samples of each, I'm collecting an example If I walk past you it's because I've already collected one that looks like yours.

Liz asked about the order in which Corinne had placed the seven small white boards on the chalk tray. Corinne had been thinking about what it was she wanted to say about the boards as she had been collecting them and also about the order in which she wanted to say this, so she had placed the boards on the chalk tray in that order. Seven was a larger number than usual. With four or five, she generally could order them as she went along. Liz noted that if she is able to pick up small white boards in the order in which she wants to talk about them, she can put them on the chalk tray in that order. If she just picks up a board, however, she finds it hard to know where it might go on the chalk tray.

Kerry commented that this process reminded him of a game show. Visually he saw Corinne placing the small white boards up there on the chalk tray with the writing facing away from the students and it was like the next thing she was going to do would be to reveal Door Number 1. Placing the boards back side to the students builds suspense. Also they see only one white board or one group of white boards that are related at a time. This draws attention to one particular idea or concept that the instructor is trying to bring out. Doing this avoids a common problem that happens in talks when a speaker puts too much information on a slide and people are reading, reading, reading and not paying attention to anything that is being said.

Corinne began the small white board discussion by holding up two that had included the symbol of an electric field; one with V = ∫ E⋅ds and another with V = ∇E.

Corinne: Ok. One of the questions was what should you write the potential in terms of? And some of you started talking about electric fields so here we have one.

Corinne held up the white board with V = ∫ E⋅ds so all could see.

Corinne then interpreted what the student had written:

Corinne: which says that the potential is the integral of the electric field something, this looks like a line integral to me. the line integral of the electric field but since I haven't told you anything about what the electric field IS, this is probably not the answer to the question that I was looking for.

She then held up the other small white board with V = ∇E :

Corinne: Here we have actually that the potential is the derivative of the electric field and some of you may or may not be familiar with this funny del sign (∇)

Then she held up both small white boards side by side:

Corinne: So we have a vote here, it's either the integral of the electric field or it's the derivative of the electric field (pause) so we're going to abandon this question until maybe even next paradigm but if Liz will record those two possibilities, we will have that vote later.

Corinne then put both boards down on one of the tables.

While watching this segment of the video, Corinne commented that the pause was definitely a deciding-what-to-do moment, whether to have that conversation. It was definitely a decision that this was not a conversation she was trying to have that day but was trying still to acknowledge that some students were thinking about that and that that was a conversation that still needed to happen sometime.

Next Corinne turned over the rest of the boards. There were five, with a variety of symbols, including $V$ (also $P$) for potential, $Q$ or $q$ for charge, $r$ for distance, and $k$ for a constant:

$$\frac{kQ^2}{r^2} \qquad\qquad Q_1 Q_2$$ $$\frac{kQ}{r^2}$$ $$P = K \frac{q_1 q_2}{r^2} \qquad\rightarrow\qquad \hbox{no 2nd charge}, \qquad\hbox{Potential}=0$$ $$V = \frac{Q}{4πε_0 r}$$ $$V = \frac{1}{4πε_0}\; \frac{q}{r} \hat r$$ Corinne: So we have a selection of answers here that I want to discuss a little bit.

Pointing to the various small white boards, she compared the students’ responses:

Corinne: So we have two people, three people saying that there's an r squared in the denominator and two people saying there's an $r$ in the denominator

We have one person claiming that the potential is a vector (points to board) and another person claiming that the potential is a scalar (points to adjacent board)

We have two people, three people, claiming there's a single $Q$ in the numerator and we have two people claiming that there are two $Q$'s or perhaps a $Q$ squared in the numerator

What do you have to say about these choices?

Kerry wondered why Corinne was telling the students rather than asking them to compare the responses on the small white boards. If she was trying to establish a pattern for how the students behave in class, he thought that asking them to do the comparison would have been useful. Corinne agreed but noted that some of the students might not have been able to read some of the boards where the writing was so small and she probably was trying to speed things up. She commented that she was probably spending twenty minutes here on this activity which in the past she might have spent 30 seconds on, writing $V= k \frac{Q}{r}$, and saying this is a potential due to a point charge, here's the answer.

Corinne noted that as the teacher in the classroom, one is constantly having to make choices about how long something is taking versus what the students would get out of taking more time. The five small white boards showed the three things that she was expecting: the difference between scalars and vectors, how many $Q$s were in the numerator, and how many factors of $r$ were in the denominator. So what she did was just point those things out to the students, focused their attention on differences among the responses on the small white boards, and then asking for comments. That was a procedure that she typically used for this particular kind of small white board question.

A student responded immediately by pointing out that the expression written on one of the small white boards was for a force rather than potential:

Student: Isn't the one on the far left the force? between two point charges?

Corinne repeated the question while picking up and holding high the board with $k Q2/r2$ so all could see it:

Corinne: Isn't the one on the far left the force? What force?

Student: A point charge on another point charge?

Corinne turned to the whole group and asked:

Corinne: What do you think of that answer?

There was general agreement:

Students: Yeah

While watching the video of this interchange, Liz pointed out that the student’s question had come out easily, that Corinne had posed a question to the class than might have been viewed as rhetorical but the student immediately answered with a question; it seemed like a question asking norm had been established. It sounded like a genuine question, the student was not going to commit to 'that's the force.' Corinne asked the rest of the students for their perspective and everyone in the class said yes.

Corinne: Yes, what people tend to lodge in their head is the formula for force and not the formula for potential and so when I ask this question, out come little pieces of the force law as opposed to the potential law.

By acknowledging that students typically remember the force law, Corinne eased the anxiety that students might have been feeling if they had responded in this way. Then she moved on to make explicit why this response was not appropriate in this case:

Corinne: All right. So this is the most typically memorized and of course if you talk about the force, you have to have two objects in the universe Corinne walked over to a student and put her hand on his shoulder to demonstrate vividly that a force involves an interaction:

Corinne: Excuse me (name), If I want to have a force, I have to be pushing on somebody. All right. I can't exert a force if I'm the only thing in the universe.

Corinne held up the ball again to represent visually the situation they were discussing:

Corinne: So if there's only one charge in the universe then we can't be talking about forces yet, which is why we're going to be talking about forces later on when I allow there to be two charges in the universe.

Having addressed the reason why the formula for force was not appropriate here, Corinne pointed out the implication, that the symbol Q for charge should only appear once in the formula for potential:

Corinne: All right. So we have only one charge in the universe The two charges, either a Q squared or a Q one and a Q two, come from thinking about forces.

Potentials, there's just one charge

Corinne held up the ball again to emphasize the situation:

Corinne: So there's only one factor of Q there.

In reflecting about this segment with Kerry, Corinne raised a question related to his earlier query, why had she not asked a question, why was she answering rather than asking about forces. Kerry had been thinking about the students’ thinking rather than Corinne’s teaching but noted that there is always the danger of getting into gridlock in the classroom when one asks too broad a question. There is a balance between how broad a question one wants to ask and how much guidance one gives.

Corinne agreed and noted that helping faculty to learn that point of balance was something that needed to be addressed. She was making a particular choice here that came from several years of experience with this question, that students remember the force law and not the potential law. She thought that just telling them would accomplish what she wanted to accomplish. Kerry also noted that asking students too much might induce them to start searching for what they think the instructor would want them to say and that could short circuit the classroom dynamic the instructor might be trying to set up.

At this point, one of the students asked a question about a procedure she was remembering:

Student: Isn't there something about integrating from zero to infinity?

Corinne: Isn't there something about integrating from zero to infinity? Integrating what?

Student: The potential. No. Integrating the electric field from zero to infinity.

Corinne went over to a table and picked up the white board with V = ∫ E .ds that she had discussed earlier:

Corinne: Yes there is but since we don't know what the electric field is, we can't use that fact; yes there is such a thing.

Kerry wondered why the students were so focused on the electric field, whether this was from their introductory class or something Corinne had already done in the class. He recognized the need to say “you have to wait for that,” but expressed regret about situations when it seems necessary to postpone talking about questions that students themselves have generated.

Corinne described for Kerry the evolution of the faculty’s decision to start with potentials rather than fields. Students seemed to have a lot more understanding of electric fields rather than potentials, just like their understandings about forces compared to energy. It seemed to be a question of what they were taught first and also what they had spent the most time on. The faculty had made a deliberate choice to talk about potentials first rather than electric fields to balance the reverse experience from the introductory courses.

One of the things Corinne hoped was happening was getting the students to build a network of understandings about potentials that did not involve electric fields, while still trying to say that reasoning about electric fields would be important. Her thought was that once students have this robust network for potentials, along with the already existing understandings about electric fields, with bringing the two together, they could snap these two networks into place like puzzle pieces and have a bigger network that would include both concepts. In contrast, starting with electric fields and then trying to get the students to learn about potential would involve pulling them away from a strong network to add on concepts about potentials; the students likely would just keep reusing the electric fields network of ideas that they already had and it would be really hard to pull them away.

Corinne acknowledged that this way of thinking about what was happening needed to be researched. Kerry thought there probably was cognitive science research on trying to introduce an isolated new substantial concept versus trying to bridge from an existing network. Emily noted that this contrasts with the constructivist point of view of building on what is already there and expanding and/or refining that; instead Corinne was describing setting up a new node and merging nodes rather than enriching an existing node. This is an example of a question about learning that could have deep implications for instruction if appropriately researched.

Video Clip

A student offered an analogy as a way to think about potentials:

Student: Way I think about it is to relate the electric potential to the gravitational potential because they're pretty much the same thing, the force equation is the same, so you do like force over, force times the distance? I mean that’s work

Corinne asked him to write the equation on his small white board to assist him in communicating his thinking both to her and to the other students:

Corinne: Write me down your equation so I can hold it up

The student wrote W = F⋅d and then V = ∫ K Q1Qd/r2 from 0 to d

Corinne reached for the white board, moved away, and then returned the board to the student who added dr to complete the integral expression. Corinne then took back the white board and addressed the class:

Corinne: Ok (name) is claiming that you can use the gravitational analog, and for some peculiar reason many students are much better at reasoning about the gravitational case than reasoning about the electrostatic case and you are absolutely right, except for an overall constant that has units in it, and a very important, physically important sign, all of the mathematics for gravitational fields is the same as the mathematics for electrostatic fields so if you can do it for a gravitational field and that makes sense to you, use that analogy. Let it help you! All right.

Corinne then held the small white board up for all to see:

Corinne: (Name)'s claim is work is force times distance. All right. And then the potential is the integral of the force times the distance but I think that's a variant of this.

Corinne reached for and held up the white board with V = ∫E⋅ds that had been discussed earlier.

Corinne: Since if there's only one thing in the universe, we can't yet be talking about forces, so there's good reasoning and good memory there going on but not what we need for this problem.

By welcoming the student’s suggestion, prompting him to write out his idea on his small white board, holding up the board for the rest of the class to see, and discussing its contents, Corinne had used a student’s writing on a small white board in the midst of the discussion to help communicate the student’s thinking to the rest of the class. Although the physics was not appropriate - his suggestion referred to forces, which could not occur with only one thing in the universe - she took care to complement the thinking - his reasoning and effort to pull relevant information from his memory.

In reflecting upon this segment of the video, Liz suggested that this question and the previous student question were both questions about “other things I know about potentials,” that this seemed like a “isn’t this also true?’ type of question. Corinne commented that this class had kept returning to the relation between the concepts of potential and electric field in ways that other classes had not, perhaps because at the beginning of this discussion she had held up the two boards, one an integral and one a derivative, and raised the question, “which was appropriate?” but then not dealt with it. She reflected that this may have been a mistake to raise a question and then not answer it, perhaps evidence of a danger of deflecting a student question. She had written for herself a rule, “don't answer a question that the students don't have,” and suggested there may be a corollary, “don't fail to answer a question that the students do have.” The students had this question in the sense that one of the students had said, “do you mean the electric field or the potential?” By holding up the two white boards at the beginning of this discussion, she had drawn everybody's attention to the issue.

Another student contributed an observation:

Student: You asked for the potential and not energy

Corinne: I asked for potential and not energy! Ah! and so, do you want to work with this?

Corinne retrieved and held up the small white board they had just been discussing with W = F⋅d and V = ∫ K Q1Qd/r2 dr

Student: No, because that's energy

Corinne: Yeah, this is energy. All right? So this is potential energy as opposed to potential and there's a difference between potential energy and potential Yes. What difference is there?

Student: Charge The unit of a coulomb.

Corinne: Hmm? Unit of a coulomb. Say more!

Student: The potential energy is the potential multiplied by the charge, coulomb Like if you had like a point charge, a test charge, in the vicinity of your main charge in the center

Corinne walked over to the supply table and picked up a small white ball. She represented this new situation visually by holding up the hand-sized ball representing the single charge and holding the small white ball nearby:

Corinne: Ok. So here's my main charge in the center and if I have another charge in the universe then what?

Student: Then you can calculate the potential energy between the two of them

Corinne suggested that the student use a small white board to write down what he was thinking so that she and the other students could better understand what was being said:

Corinne: Ok. Write down on some white board there the formula you're thinking about.

In this case, the student chose not to do so, indicating that the formula already existed on the earlier white board:

Student: Well it's pretty close to what you

Student2: It is what you said

Corinne: Pretty close to what?

Student: Isn't that what he had written down?

Corinne: Ok. So he has potential energy and what do you want to do with this?

Corinne held up the white board with V = ∫ K Q1Qd/r2 from 0 to d

Student: That's what

The student who had written the board interrupted by responding:

Student 1: Oh ah, you get rid of the second charge I think if that's energy, ah, that's (?)

Corinne: Yes, so the force requires both charges to be in there, right? But in the end, so this will give you a calculation for the energy and at the end of the day if you divide by the second charge (points to Qd symbol) or if you just divided by the second charge before you did the calculation

Corinne began confirming the difference between potential and potential energy but was interrupted.

At this point, Student 1 offered yet another thought, which prompted Corinne to begin redirecting the conversation.

Student 1: I was thinking about the power is equal to the current times the voltage and using that as an analogy

Corinne: Oh we're going to go to the power, ok, We're getting pretty far astray if we're headed to power I want to go back to these formulas

Corinne turned to the blackboard and pointed to the set of small white boards lined up along the chalk tray. However, Student 1 persisted in trying to explain his idea and asked for confirmation:

Student 1: I mean as far as the units of Matt’s r squared, I was getting at, because power is joules per sec and current is coulombs per sec, right?

Corinne commented on the increasing complexity, commended the process of trying to sort out what one is thinking, and explained her intent to focus on one particular aspect:

Corinne: Yeah, now we've got currents and everything Let's go back, I What you're trying to do is build a wonderful web of connected ideas in your head. You have a wonderful web of connected ideas in your head and I'm just trying to take one piece of it now.

Holding up the hand-sized ball, Corinne then restated the original small white board question:

Corinne: So, let's go back to here I want a formula in terms of the charge because that's what I gave you, no currents, no power, no forces, no electric fields.

There's one charge in the universe and what we know is the charge on the charge

Corinne continued holding up the hand-sized ball and picked up the probe for the voltmeter and held it up as well.

Corinne: If that's all we know and we're trying to find the potential I want the formula in terms of the charge.

Then Corinne directed attention to the small white boards on the chalk tray and discussed their status:

Corinne: We've got some examples here We decided it couldn't be this one because it's got a Q squared in it. She moved that board away from the rest.

Corinne: We decided it couldn't be this one because it's got a Q one Q two in it.

She moved the second board also away from the rest.

Corinne: So now we're down to three choices.

This is a good illustration of Corinne trying to monitor and control the flow of the class discussion to bring out the main points that she would have been lecturing on if this had been a lecture. This discussion was not intended to be a free-flow exchange of ideas about whatever conversation the students were interesting in having; Corinne had an agenda that she wanted to accomplish via discussion by the end of the class.

In reflecting upon this segment of the video, Corinne commented that she had clearly felt that the conversation was going off on a side path and had gone on longer than she was comfortable with so she was trying to bring the conversation back to the point she wanted to make. The student, however, was so excited about being able to articulate his reasoning that he was not letting that happen.

There was a definite sense of frustration in Corinne’s voice but she was making several different moves to try to bring the conversation back. First she had tried just stating what she wanted to do. And when that did not work, then she had tried describing what the student was doing, praising him for it, and then stating more clearly what she was trying to do. She was not just redirecting this one student’s attention but the whole class’ attention to her original question.

The boards that Corinne had discussed so far were the ones that had not responded to the question as she had intended but had brought in physical quantities other than the charge. She noted that there is an epistemological game in physics, which is that you answer a question or build a model using only the information that you have been given in the problem. For these first few boards, the students had not been playing that game. She had used those boards to try to describe the game to the students. Her intent now was to start discussing the boards of the students who had played that game.

Corinne had modeled using physical reasoning to eliminate several of the boards, such as those that mentioned are two charges when there is only one charge in the universe. She had narrowed the choices down to three. These were the three choices that she had originally expected to have come up. She had been looking for them explicitly when she was walking through the class while the students were writing the boards. At this point, they were only now starting to have the conversation that she had planned.

Corinne: So now we're down to three choices

What do you have to say about these three choices?

A student quietly proposed a reason for eliminating one of the boards. Corinne reiterated her rationale with emphasis.

Student: The potential is a number so the vector one is out

Corinne: The potential is a number.

Corinne picked up the small white board with vector notation and moved it away from the rest .

Corinne: Forces are vectors, electric fields are vectors, potentials are scalars My voltmeter is a pretty good voltmeter; it only reads a number; There is no arrow associated with it.

Corinne turned to the last two small white boards and first discussed what appeared to be different constants.

Corinne: All right. So now we have a choice K (pointing to left white board) or one over four pi epsilon naught (pointing to right board) as the constants, that's just a question of what system of units you're using. We will indeed be using the system of units where k is one over four pi epsilon naught so these two are identical in that sense.

Then Corinne directed attention to the difference in the relationships to the distance by first pointing to the 1/r2 on the left board and then to the 1/r on the right board.

Corinne: All right. So one over r squared or one over r.

Students: One over r

Corinne: One over r, why?

Student: Because the potential is equal to the integral of the electric field over some distance so that's basically saying that the electric field, which is k Q over r squared times a distance r and r's canceled and you're left with k Q over r

Corinne: I liked everything you said until you said the 'r's cancel'

Student: Oh, sorry (laughter)

Student: units

Corinne: You said really beautiful things until you said it was the integral, and then you said, the r's cancel If you're integrating

Student: I meant after you integrate over a certain distance, the r is just the distance between so

Corinne: You integrate something with an r squared in the denominator, dr, You don't get to cancel the r's, (oh) You have to do the integral of r to the minus two

Corinne then wrote on the board while stating what she was writing:

Corinne: The integral of r to the minus two is r to the minus one (writes ∫ r-2 dr = r-1 ) Which it is but it's not because you cancelled the r's

Student: Seems that way

Corinne: But absolutely, one of these is the integral of the other and that's what we've been discussing One of these is the force or the electric field, both go like one over r squared, you integrate that (pointing to board), you get a potential and that gives you the one over r; So this is the potential

Corinne held up the board with V = Q/4πεor

In reflecting on this segment of the video, Corinne commented that this was an example of a totally unexpected student error, which is the sort of thing that happens to you when you let a student ask questions. She did not have a good answer for it and what she offered was proof by intimidation. She had no idea what else to say to him.

Liz wondered why Corinne had objected to that so strongly. Corinne noted that the student was just doing that the work was force times distance and the distance was r and the formula had a one over r squared and the r's canceled, rather than taking the integral of one over r squared dr; that these are not the same mathematics. Liz commented that she often thinks of an integral expression as multiplying the units of the integrad with the units of the integration variable, that thinking about dimensions is a productive way of thinking. Corinne interpreted the student’s statements as not talking dimensions but that he was making a calculus error that needed to be fixed.

Having finally established the formula for the electrostatic potential due to a charge that is the only thing in the universe, V = Q/4πεor, Corinne directed attention to what the symbols meant. For her, an answer in terms of symbols is not all there is to an answer. One also has to understand the symbols:

Corinne: Now, what do we mean physically by the r here?

Student: Radius

Corinne responded to this ‘typical’ meaning of r by holding up the ball and asking:

Corinne: Radius. Of the charge

Multiple students responded:

Student: How far away you test the charge Student: The distance from the charge Student: Distance between the center of the charge Corinne: Hmm? Between the center of what?

Student: The charge and your observation point

Corinne noted that some questions you get a flurry of voices and as a faculty member you have to choose to which ones you are going to respond.

Corinne: Where's my observation point?

Student: Wherever you put your probe

Corinne picked up the probe, held both it and the ball representing the charge up so all could see. Corinne was using the probe and ball to make the meaning of the symbol r vivid.

Corinne: Ah. the distance between here (ball) and here (probe). All right. The distance between here and here. It's a distance.

Video Clip

A student asked a question involving a more nuanced expression for the distance between the charge and the probe:

Student: r minus r naught?

Corinne: What about r minus r naught?

Student: The distance between there and there

Corinne: Write me something on your white board. I don't know what r minus r naught means.

Corinne and the class waited and watched while the student wrote his expression on his small white board. Then she held it up for all to see:

While viewing this segment of the video, Liz commented about this use of the small white boards, that Corinne was asking individual students to write mathematical expressions on the small white boards so that the students could communicate more clearly with Corinne and so that Corinne could use the white board to communicate their shared meaning to the rest of the class. Not only can an instructor ask small white board questions to the entire class but one can also ask them of individuals to help clarify their meanings.

Corinne agreed that when a student asks about a formula, she and the student can communicate much more clearly if the student writes the formula on a small white board. She noted that this is what professionals do when talking with each other, they write on napkins or backs of envelopes or whatever board is nearby to be sure that they understand and agree on the formula that they’re discussing.

Corinne: Ah. Ok! He's trying to write a magnitude of r minus r naught. So where is r and where is r naught?

Student: r naught is at the origin

Corinne: Where is the origin?

The student pointed at a representation of a coordinate system, the three dowel rods connected at right angles, that was sitting on a table in front of Corinne.

Student: That.

Corinne put down the student’s white board and while still holding up the ball representing the charge, held up the coordinate system so all could see it. She then put both the ball and the coordinate system down together on the table.

Corinne: Ok. So I'm going to put this charge at the origin. Imagine it's in the center. All right. And then?

Corinne picked up the student’s white board again and held it up for the students to see.

Student: The r is wherever you’re thinking about at the moment

Corinne walked back to the table and picked up the probe and held it high for all to see:

Student: wherever that is

Corinne: Ok. All right. If I put the charge at the origin, then what does that tell you about this?

She pointed at the r in r – r’ on the student’s white board:

Students: r naught is zero

Corinne: Then it's just zero. What kind of a zero? It's a zero vector. All right. So the zero vector minus r prime is just the vector from the origin (points to coordinate system on the table) to here (picks up probe).

In discussing this video, Corinne noted that in a discussion in which she was trying to help the students understand which is r and which is r prime, that she had mixed it up herself. So the words “zero vector minus r prime” should have been “the zero vector minus r.” Fortunately the rest of the discussion was correct and she hopes she did not confuse anybody too much.

Corinne: So yes indeed, if I put this charge at the origin (points to coordinate system), then this r here (points to white board with correct formula V = Q/4πε0r) is just the distance from here (ball representing charge) to here (voltmeter probe), the distance from here to here.

In viewing this segment of the video, Corinne commented that she was trying to get the students to see the difference between scalars and vectors and by manipulating the physical things, trying to get them to focus on the geometry.

During this conversation about r minus r naught, a webcam videoing group 6 shows a student sitting quietly until Corinne says “write on your white board” to the student with whom she is conversing about r minus r naught. The student shown on Webcam 6 turns to his white board in response even though the comment was not directed to him.

Visible in the video was the student’s initial response on his white board to Corinne’s planned small white board question, what is the electro potential due to a point charge? When Corinne initially posed this question, the student had drawn a circle, put a question mark in the circle, drawn an arrow pointing to the circle, and after a long pause while thinking, finally written kQ/r2. He also added some words (that cannot be discerned on the video.) Throughout the long discussion of the various student responses that Corinne had facilitated, he had not changed anything on his small white board.

However, now in response to Corinne’s direction to another student “write on your white board,” this student took his cloth eraser and carefully erased the 2 from the r squared. Then he also revised his drawing by extending the line to the center of the circle and labeling the line r.

This spontaneous correction by a student on what he had written earlier on the small white board illustrates another advantage of these devices, that they can provide a prominent visual image of the focus of a discussion for each student, one that the student can revise later as needed.

Having clarified the simple case with the charge placed at the origin, Corinne then picked up the ball representing the charge and moved it away from the coordinate system:

Corinne: What happens if I choose not to put this at the origin?

A student responded, describing the distance between the probe and the charge while moving her hands apart to demonstrate that distance.

S: (?)

Corinne moved back toward the blackboard to point to the r on the small white board on the chalk tray, the one with the correct formula for the electrostatic potential, V = Q/4πε0r.

Corinne: Yes, what I mean here is always the distance, whether or not I happen to choose my origin to be at the center of the charge.

Corinne again held up the ball representing the charge and the probe to demonstrate the distance between them that was the focus of attention.

Corinne: Now if I have only one charge in the whole universe I would be really stupid not to put the origin where the charge is.

Students: (laugh)

Corinne: (laughs), Ok. That would be really stupid so always put the origin some place nice. All right.

Then Corinne walked over to her supply table and picked up a basketball.

Corinne: But as soon as I let the universe be more complicated so that there are two charges in the universe, I can't put them both at the origin, because they're separated

Corinne held both balls up to show two charges separated by a distance. Then she moved the ball representing the initial charge back down next to the coordinate system on the table:

Corinne: I can put one at the origin maybe All right

But then she again held up the ball representing the initial charge in one hand and the basketball representing the second charge in the other hand.

Corinne: but as soon as I have two charges in the universe it's not so simple to say that this r (points to r on the small white board with V = Q/4πε0r) represents the distance between the origin (points to coordinate system) and this point (picks up voltmeter probe) It represents something else. So now, imagine that the second one

Corinne then gave the basketball to one of the students to hold.

Corinne: Thank you. Ok so now we have two charges in the universe. All right. And now we have my potential meter.

Corinne held up the probe and the ball representing the initial charge

Corinne: Let's look at just the potential due to this one but now I can't put it at the origin So how do I talk about the distance between here (ball) and here (voltmeter probe)? How do I write it down?

While watching this segment of the video, Corinne commented on how much effort she was putting into this issue, that some students write down symbols for the magnitude of r minus r prime but have no idea geometrically what those are referring to and that she keeps trying to find ways to get everybody in the class to understand that.

Corinne paused. In the video she can be seen bending forward to look at what one of the students was writing and drawing on her small white board at a table nearby. Meanwhile another student responded verbally:

Student: Magnitude of the difference between the vectors

Corinne: Magnitude of the difference between the vectors

The student writing and drawing then held up her small white board to show Corinne.

Student: (?)

Student: Could have one at the origin

Corinne. I could have one at the origin

Corinne took the white board and showed it to the class.

Corinne: All right. So you want me, You're going to make me work really hard for this answer

Corinne accepted this student’s offer of an arrangement that would be useful under certain conditions, used the dowels representing the coordinate system to make the suggestive vivid, and then articulated those constraints.

Corinne: So if you're really clever, you can put one at the origin and you can put the other one along the x axis (picks up coordinate system)

Right? So then you can just use a scalar distance there (points to board)

But do I have to put my probe also on the x axis somewhere between them?

Student: Well I

Corinne: What if I want to measure up here? (holds probe up high)

Another student explained the situation if there were only one charge:

Student: If you're working in a three dimensional space, it creates a potential around itself. So if you go out from it at any radius in 3-D, you get the same potential.

Corinne accepted her suggestion also, made it vivid by holding up the probe to represent measuring the potential anywhere in space around the charge, and then articulated the constraints in system with more than one charge.

Corinne: Right. If I go out anywhere around this one (holds up probe) the same radius, I get the same potential. Absolutely.

But as I go probing around here (moves probe around) and I'm getting the same potential from this one (points to ball), I'm not staying the same radius from that one (points to basketball)

Corinne: So all I want is the mathematics of how I describe the distance between here (probe) and here (ball on table).

Another student contributed a thought:

Student: The magnitude of the distance (?)

Corinne: Exactly. It's the Star Trek example.

A student’s spontaneous and unexpected contribution prompted this interaction among the instructor and several students. The student had used the resource of a small white board to express what she was thinking by sketching a diagram, the sketch made her thinking visually available to the instructor who leaned over to see what the student was drawing, the student was able to offer her thinking via the small white board to the instructor who then was able to convey and discuss the student’s ideas with the whole group; several members of the whole group then contributed to the conversation, and made explicit the connection between the current discussion and the activity in which the students had participated earlier in the session.

S: (?)

At this point, Corinne returned to the student who was the author of the initial whiteboard and asked him to fix what was written there by indicating that the r and r’ were vectors:

Corinne: All right which is what (Name) was trying to write down here and the only quibble I really have with you is “these are vectors” (takes board over to student and points at board) (write vectors on the bottom?) Ok?

Then Corinne summarized what the meaning was for the various position vectors involved:

Corinne: So what I have to do is say “here's the origin” (points to coordinate system) there's a position vector from here to here (points from origin to ball); there's a position vector from here to here (points to origin, holds up and points to probe); and if I want the distance between these two places (the probe and the ball), I take the difference between those two position vectors and I take the magnitude thereof and it looks like this (points to blackboard)

Corinne then reviewed what they had done that day and why:

Corinne: That's why we played Star Trek and as we get more and more charges in the universe and get up to the complexity of something that's actually happening in the laboratory, we're going to need to be using an expression like this (points to blackboard)

Corinne: ( to student) keep your board for Friday