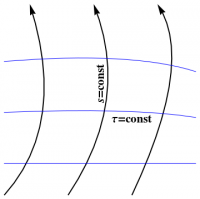
Consider a family of geodesics, with velocity vectors of the form \begin{equation} \vv = \dot{\rr} = \Partial{\rr}{\tau} \end{equation}

Figure 1: A family of geodesics.

as shown in Figure 1. Since these curves are geodesics, their velocity vectors satisfy \begin{equation} \dot{\vv} = 0 \end{equation} Label the geodesics by some coordinate(s) $s$, and consider the separation vector \begin{equation} \uu = \rr' = \Partial{\rr}{s} \end{equation} between nearby geodesics. By construction, we have \begin{equation} \dot{\uu} = \vv' \end{equation} We are interested in the behavior of the separation $\uu$ along the geodesics, and we have \begin{equation} \ddot{\uu} = \left(\vv'\right)\Dot = \left(\vv'\right)\Dot - (\dot{\vv})' \end{equation} where we have used the fact that $\vv$ is a geodesic in the last equality. This expression involves second derivatives of $\vv$ in two different directions, and changes sign if those directions are interchanged. It should not be surprising that this combination of derivatives is related to $d^2\vv$. As shown in the next section, it turns out that \begin{equation} \ddot{\uu} = -d^2\vv (\uu,\vv) = -\Omega^i{}_j(\uu,\vv) v^j \ee_i = -R^i{}_{jk\ell} v^j u^k v^\ell \ee_i \end{equation} and we can think of this as \begin{equation} \ddot{\uu} = -R^i{}_{\tau s\tau} \ee_i \end{equation} The $s$-component of this expression is indeed $-R^s{}_{\tau s\tau}$, as claimed in the examples in the previous section.