In § {Activity: Using Technology to Visualize the Divergence}, you were asked to apply the geometric definition of divergence. It is important to realize that the divergence is a function; it is not enough to examine the behavior near a single point, such as the origin.

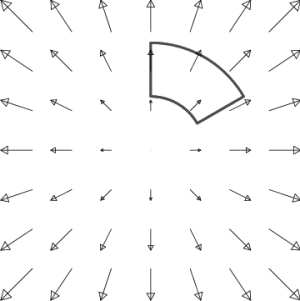
It is helpful when trying to apply the geometric definition of divergence as flux per unit volume to draw a box of the appropriate shape. If the vector field is round, use round boxes! An example is shown in Figure 1.

Most of the given vector fields were drawn in 2 dimensions, although they are in fact 3-dimensional. There is also a 2-dimensional notion of divergence as “flux per unit area” which can be applied to vector fields in 2 dimensions, but be aware that this 2-dimensional flux has different dimensios than the 3-dimensional flux.