Models for Water

A large number of 'hypothetical' models for water have been developed in order to discover the structure of water (for reviews of their development see [275], for a review of their use in supercritical water see [433] and for an appraisal of their accomplishments see [400]), on the basis that if the (known) model (i.e. computer water) can successfully predict the physical properties of liquid water then the (unknown) structure of liquid water is determined. They involve orienting electrostatic effects and Lennard-Jones sites that may or may not coincide with one or more of the charged sites. The Lennard-Jones interaction accounts for the size of the molecules. It is repulsive at short distances, ensuring that the structure does not completely collapse due to the electrostatic interactions. At intermediate distances it is significantly attractive but non-directional^a and competes with the directional attractive electrostatic interactions. This competition ensures a tension between an expanded tetrahedral network and a collapsed non directional one (e.g. similar to that found in liquid noble gases). Generally each model is developed to fit well with one particular physical structure or parameter (e.g. the density anomaly, radial distribution function or the critical parameters) and it comes as no surprise when a model developed to fit certain parameters gives good compliance with these same parameters (e.g. see [984]). It is also the case that, in spite of the heavy computational investment in the calculations, the final agreement (or otherwise) with experimental data is often 'by eye' and not statistically tested or checked for parametric sensitivity. Also, tests for 'fit' often seem to be completed with publication in mind rather than rigor; thus many papers use the radial distribution fit with diffraction data as their 'gold standard' in spite of the major fitted peaks (where the agreement looks so impressive 'by eye') being derived from the tetrahedral nature of water that is built into every model and overpowering any disagreement in the fine detail. Also unfortunately, the purity, isotopic mix and perhaps even the ortho/para spin state present in real water may cause difficulty over choice of the value of the physical parameter [400], as models only utilize one isotopic form and ignore the spin state and the presence of other entities such as ions. Also, there is still disagreement over which value of some physical parameters to use, e.g. for the dipole moment. Whether model results agree with other physical properties of water then acts as proof (or otherwise) of their utility. By and large, the more fitting parameters that are required by the model (and some require over 50), the better the fit. Some models show a lack of robustness due to their sensitivity to the precise model parameters [206], the system size or the calculation method [619, 649]. A study of sensitivity of water's behavior with respect to changes in the parameters using the TIP4P model potential showed that σ, followed by the O-H bond length, had the major effects on the density, enthalpy of vaporization and radial distribution function fits [494]. A separate sensitivity analysis showed that the thermodynamic properties of water models were most sensitive to the van der Waals repulsive, the short range Coulomb and the polarization components of the potential [1042].

It can be noted that a number of these models use water molecules with a wider (tetrahedral) H-O-H angle and longer H-O bond length than those expected of gaseous or liquid water and indicative of the importance of including parameters giving strong hydrogen bonding. Water molecules in liquid water are all non-equivalent (differing in their molecular orbitals, their precise geometry and molecular vibrations; for an extreme case see the water dimer) due to their hydrogen bonding status, which is influenced by the arrangement of the surrounding water molecules. Some models are polarizable [867] to make some allowance for this,^c,d whereas other simpler models try to reproduce 'average' structures.

A recent review listed 46 distinct models [400], so indirectly indicating their lack of success in quantitatively reproducing the properties of real water. They may, however, offer useful insight into water's behavior. Some of the more successful simple models are opposite with their parameters given below. Models types a, b and c are all planar whereas type d is almost tetrahedral

¹ Average values; ² Polarizable models; ³ charge = -2.48856; ⁴ Buckingham potential^a, This exponential potential presents a more flexible (i.e. softer) surface compared with the Lennard-Jones r^-12 interaction.; ⁵ with charge on oxygen atom; ⁶σ and ε are Lennard-Jones parameters. The separation and depth of the potential energy minimum between two similar molecules (equivalent to diameter); ⁷ has charges on the lone pair sites (_L) as in model type d and the mid-point site (_M) as in model type c; ⁸ has only a single, center of mass, interaction site with a tetrahedrally coordinated sticky potential that regulates the tetrahedral coordination of neighboring molecules; ⁹ a polarization charge q_pol (-8) is connected by a spring to site q₂, the total charge (q_pol+q₂) being given in the table as q₂; ¹⁰ The charges are smeared (i.e. not point charges) using Gaussian distributions with widths of 0.455 Å and 0.610 Å for q₁ and q₂ respectively; ¹¹ Zero potential position at 3.25 Å. ¹² This model over-structures the water. ¹³ A 'Drude' particle carrying a negative charge -1.71636e is attached by a harmonic spring (4184 kJ mol^-1 Å^-2 while the oxygen carries a charge +1.71636e. ¹⁴ Induced dipoles are placed on the atoms. The van der Waals terms give a deeper, steeper and more distant energy minimum (-1.187 kJ mol^-1 at 3.726 Å) than the typical Lennard-Jones potential. [Back] 

Some of the above values are varied slightly by different workers. Other workers use diffuse electron density [203] or polarizable versions of the non-polarizable models, using flexible bonding (e.g. SWFLEX-AI),  induced dipoles (e.g. [181]), energy optimization (e.g. the TIP4P-FQ version of TIP4P) or movable charge (e.g. SWFLEX-AI), all of which generally give better fit but at a significantly increased computational cost [198]. Polarization mutually strengthens the hydrogen bonding and partially compensates for the absence (except statistically) of the known long range interactions and the dependence of these models on short-ranged forces. Diffuse electron density [203] varies the effective charges with distance. Such models generally perform better away from the ambient conditions under which they are parameterized than the simpler models.

Some of the calculated physical properties of the water models.

Many of the data values given in the table vary significantly between different workers (see e.g. [185]). A comparison of some of the properties of the gas phase dimers for various models are given in a recent paper [704].^b As can be deduced from the data given (and other data), although such simple models are of great utility, no universally applicable model can be identified at this time. It should also be noted that many simulations are performed with just a few hundred water molecules within rectangular periodic boxes no more than 2.5 nm along each edge for times equivalent to a few picoseconds; conditions that reduce discovery of long-range effects and introduce artifacts. Use of cut-off lengths (even long ones) in the intermolecular interactions may also introduce artifacts [761]. It should be noted that there is a strong correlation between the length scale of any water structuring and the time scale which is required to see it. The predictive value of water models has been questioned [202] and, even with current developments, their general application should be approached with caution [203]. Clearly the water molecule is a flexible molecule with electronic polarization and models that do not include both these characteristics together with their interactions are unlikely to be good predictors. 

The agreement of the icosahedral cluster model of water with the O···O radial distribution function is in marked contrast to the use of many polarizable and non-polarizable models for water, which do not show any fine structure. The popular TIP4P model underestimates the tetrahedrality of the water molecule's environment, which explains its poor estimate of the dielectric constant. It is, however, remarkably good at qualitatively describing water's phase diagram [669] and this has been developed further in TIP4P/Ice [838]. The SPC/E, PPC, and TIP4P, [3] and BSV, CC, DC, SPC/E and TIP4P, [93] models are reported as failing to properly describe the experimental O···O radial distribution function. The TIP3P and SPC show particularly poor agreement, the TIP4P, SPC/E and PPC show improved agreement but the recent models TIP4P-FQ and increasingly used TIP5P give further improvement [199] at a computational cost. The popular models SPC, SPC/E, TIP3P and TIP4P produce poor agreement with water's melting point (giving melting points of 190 K, 215 K, 146 K and 232 K respectively) and SPC, SPC/E, TIP3P and TIP5P do not give ice1h as a stable phase, replacing it with ice II [775] or improbable unrealistic crystal structures and many models mistakenly predict antiferroelectric character for the ordered phases of ice (e.g. ice XI) [1051]. Different models also give very different lowest energy structures for small water clusters [857]. It is also true that models for liquid water bearing little relationship to reality (e.g. involving only two dimensions or 8-molecule cubic arrangements) can be used to calculate similarly close results for a small number of water's properties. Nonpolarizable models have been shown to be inherently unable to simultaneously predict certain physical properties, such as melting temperature and the temperature of maximum density, whatever parameter values are chosen due to the limited number of variable parameters [1079]. None of 40 rigid, flexible, polarizable and ab initio models were capable of simultaneously agreeing with both the experimental radial distribution model and the experimental internal energy [245]. Serious discrepancies, concerning the first coordination shell hydrogen bonding, have been noted between the molecular dynamics simulations using these models and X-ray absorption spectroscopy [613]. Other modeling studies have failed to reproduce parts of water's vibrational spectrum even qualitatively [696]. Artifacts, such as phase transitions, may be unexpectedly produced in water simulations [1056] or, worse, go unnoticed. More complete agreement may require many-body parameters [221] as three-body effects have been shown to contribute 14.5% (or more [728]) to the internal energy and these cannot be properly represented by potentials that distort two-body effects [465]. A recent model (GCPM ) using polarizable smeared charges, rather than the other models' point charges, has however shown considerable promise [859]. Other recent steps forward are the inclusion of quantization, which is shown to have significant consequences on water's structuring [863], and the need for high order multipole components, up to hexadecapole, in order to achieve the correct ferroelectric structures for the ordered ice phases [1051]. In a study attempting to combine diffraction, infrared and x-ray absorption data, it was concluded that current water models show poor fit [1159]. Altogether, it is clear that in spite of water appearing to be a very simple molecule, it remains very difficult to model realistically.

In the light of these observations, it is unsurprising that contemporary water models are relatively poor predictors for the conformation and hydration of biological molecules in solution (e.g. [596]) and it may be useful to develop water models specifically for use with biomolecular solvation.

^a Note, however that water is not a spherically symmetrical molecule as judged by the variation in the van der Waals radii [206]. Also, in these models the Lennard-Jones interaction exerts a repulsive effect on hydrogen bonding whereas some report it is attractive [548] even at this close contact. The Lennard-Jones potential is made up of a twelfth power repulsive term and a sixth power attractive term:

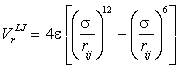
Shown below right is the Lennard-Jones potential for the SPC/E model (solid red line). The σ parameter gives the molecular separation for zero interaction energy. The minimum energy (-ε) lies 12% further at σx2^1/6 Å.

Also shown (dotted blue line) is an equivalent Buckingham potential (σ 3.55 Å, ε 0.65 kJ mol-1, γ 12.75); the σ parameter in the Buckingham potential gives the σx21/6 position in the Lennard-Jones potential.      [859] [Back]

^b Models may be checked for agreement with gas phase clusters (e.g. water dimers) before use in liquid water simulations. Such compliance, however, should not be a necessary prerequisite for accurate liquid water predictions. [Back]

^c Molecular polarization may be electronic (caused by the redistribution of its electrons), geometric (caused by changes in the bond lengths and angles) and/or orientational (caused by the rotation of the whole molecule) [867]. This paper [867] describes Charge-On-Spring polarizable force fields (e.g. COS/G3) as most suitable for aqueous solutions of proteins. Alternatively, a model possessing out-of-plane polarization and fluctuating charges (POL5/TZ) is proposed best for comparison with experimental vibrational data [878]. [Back]

^d One model describes the water molecule solely in terms of dipoles and polarizabilities on the atoms and a quadrupole on the oxygen atom. [736]. [Back]