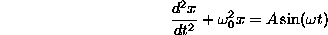Differential Equations
Differential equations are equations that relate a function f to its
derivatives. This type of function finds widespread application in science and
technology ...
from Encyclopedia Britannica
To classify differential equations we use a variety of terms, the most usual
ones being,
- ordinary - partial
- A differential equation is called ordinary if the unknown function and its
derivatives depend only on one independent variable. In a partial differential
equation the unknown function and its derivatives depend on at least two
independent variables.
Example:
The time-dependent Schrödinger equation,

is a partial differential equation as it contains two independent variables
x and t.
- order
- The order of a linear equation is determined by the order of the highest
derivative in the equation.
Example:
The equation describing the harmonic oscillator,
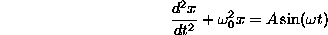
is a second-order differential equation as the the highest derivative is of
second order. Sometimes it is possible to solve a differential equation by
reducing it to one of lesser order. However, this does not change the order of
the initial equation.
- homogenous - inhomogenous
- A differential equation is called homogenous if every term in it depends on
the unknown function or its derivatives. It is inhomogenous if there is at
least one term which depends only on the independent variables or is a constant
different than zero.
Example:
The differential equation of a driven harmonic oscillator,

is inhomogenous as the term on the right only depends on t.
- linear - nonlinear
- A linear differential equation contains only linear terms of the unknown
function and its derivatives.
Example:
The differential equation describing a realistic pendulum,

is a common example.
- degree
- If a nonlinear differential equation can be expressed as a polynomial of the
unknownfunction and its derivatives, then the degree of this differential
equation is the highest power of this polynomial.
Back
to main document.