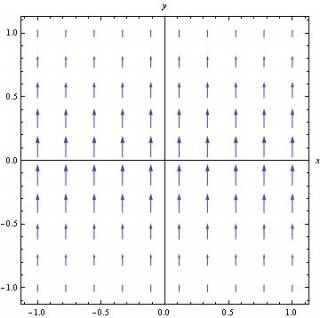
Visualizing Divergence

Highlights of the activity

1. This computer visualization activity is designed to provide students with a geometric understanding of divergence.
2. Students use Maple/Mathematica and the definition that divergence is flux per unit volume to practice estimating the value of the divergence at any point for a variety of vector fields.
3. Discussion focuses on choosing a volume that respects the symmetry of the field and on considering the divergence at many different points.

Reasons to spend class time on the activity

The traditional discussion of divergence in vector calculus derives an algebraic expression in rectangular coordinates. We prefer to give a geometric derivation as flux per unit volume through an appropriately shaped box. This worksheet helps students learn how to predict the value of the divergence at any point by looking at the vector field near that point.

Reflections

• Reflections from the original implementation.

• Reflections from "official" adapters/adopters.

• Reflections from the general public.

Instructor's Guide

• Wiki version

Maple Worksheet

vfdivergence.mw (Maple 13)

vfdivergence.mws (Maple 11 Classic)

Mathematica Notebook

vfdivergence.nb