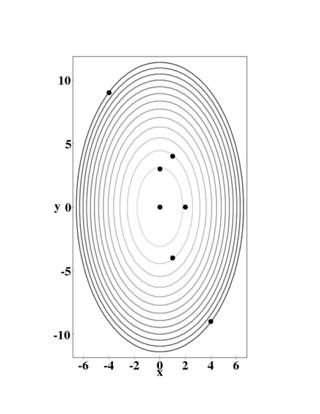
Navigating a Hill
Highlights of the activity
- This small group activity is designed to help students learn how to conceptualize the geometric properties of the gradient.
- Students are asked to find the direction and relative magnitude of the gradient at various points on an elliptical hill.
- The wrap-up discussion addresses the idea that the gradient doesn’t point toward the ‘top of the hill’ but rather, locally, in the direction of greatest rate of change. The dimensionality of the gradient is also discussed.
Reasons to spend class time on the activity
This activity helps students understand the geometric interpretation of the gradient and gives them an opportunity to practice computing a gradient and a directional derivative.