Homework for Static Fields

  1. (PathIndependence) Students explicitly compute the work required to bring a charge from infinity using two different paths. Include part (e) as an additional path that cannot be solved by integration. (You must invoke path-independence of conservative fields.)

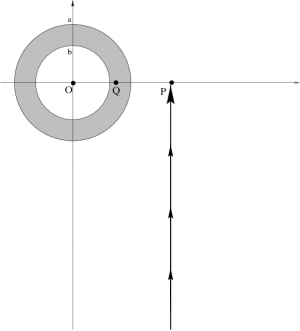
    The gravitational field due to a spherical shell of mass is given by: %/* \[ \Vec g =\begin{cases} 

    0&r<b\\

    -\frac{4}{3}\pi\rho\,G\left({r}-{b^3\over r^2}\right)\hat{r}&b<r<a\\ -\frac{4}{3}\pi\rho\, G\left({a^3-b^3\over r^2}\right)\hat{r}&a<r\\ 

    \end{cases}

    \]

    where $b$ is the inside radius of the shell, $a$ is the outside radius of the shell, and $\rho$ is the constant mass density.

    1. Using an explicit line integral, calculate the work required to bring a test mass, of mass $m_0$, from infinity to a point $P$, which is a distance $c$ (where $c>a$) from the center of the shell.

      Figure: integration paths for parts a), b), and c)

    2. Using an explicit line integral, calculate the work required to bring the test mass along the same path, from infinity to the point $Q$ a distance $d$ (where $b<d<a$) from the center of the shell.

    3. Using an explicit line integral, calculate the work required to bring the test mass along the same radial path from infinity all the way to the center of the shell.

    4. Using an explicit line integral, calculate the work required to bring in the test mass along the path drawn below, to the point $P$ of question a. Compare the work to your answer from question a.

      Figure: integration path for parts d)

    5. What is the work required to bring the test mass from infinity along the path drawn below to the point $P$ of question a. Explain your reasoning.

      Figure: integration paths for part e)


Go Back to the Static Fields Course Content Page
Go Back to the old (3-week) Static Fields Course Content Page