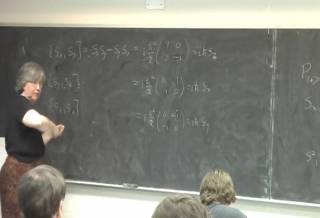Finding if $S_{x}, \; S_{y}, \; and \; S_{z}$ Commute
Highlights of the activity
- Students are placed into three different sections and are asked to determine if any of the quantum operators $S_{x}, \; S_{y} \; or \; S_{z}$ commute.
- Groups will find that none of the quantum operators commute and therefore do not share the same basis for their respective eigenvectors.
Reasons to spend class time on the activity
Showing that none of the spin operators $S_{x}, \; S_{y}, \; or \; S_{z}$ commute provides mathematical evidence for many of the properties only observed at this point in the course. Since none of the spin operators commute, none of the operators have the same base. Also, since none of the spin operators $S_{x}, \; S_{y}, \; or \; S_{z}$ commute, none of the spin operators can be measured simultaneously. This rule of simultaneous measurement shows up commonly in quantum mechanics, and showing students the properties of commuting operators in more simple cases will make applying the same concepts easier in the future.