Ampère's Law and Symmetry
Consider the magnetic field due to a uniform current everywhere in the $xy$-plane, moving in the $x$-direction. In what direction is the magnetic field? The Biot-Savart Law says that \begin{eqnarray*} \BB = {\mu_0\over 4\pi} \int {\KK(\rrp)\times(\rr-\rrp)\,dA'\over|\rr-\rrp|^3} \end{eqnarray*} But $\KK\times(\rr-\rrp)$ is perpendicular to $\KK$, and since $\KK$ points (everywhere) in the $x$-direction, the $\xhat$-component of $\BB$ must vanish, that is, $B_x=0$.
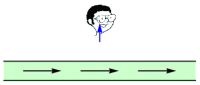
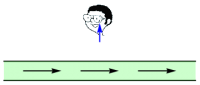
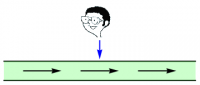
Figure 1: The symmetry argument used to show that the magnetic field points parallel to an infinite plane with a uniform current.Consider now the $z$-component, $B_z$. The Biot-Savart Law implies that reversing the direction of $\KK$ (everywhere) must also reverse the direction of $\BB$. Suppose that $B_z>0$ somewhere, as shown in the first diagram in Figure 1. Stand there, close your eyes, and spin around, and suppose you wind up facing in the opposite direction. On the one hand, the magnetic field can't change — you've moved, but it hasn't. This scenario is shown in the second diagram in Figure 1. Thus, $B_z$ must still be positive. On the other hand, you have no way of telling that you are now facing left rather than right; what you now see must be exactly the same as if the $xy$-plane had turned underneath you, reversing the direction of $\KK$, and hence also of $\BB$. By this argument, as illustrated in the third diagram in Figure 1, $B_z$ must now be negative! The only way out of this predicament is for the $z$-component of $\BB$ to vanish, that is, $B_z=0$.
Figure 2: Using symmetry to show translational invariance of the magnetic field.Furthermore, $\BB$ clearly doesn't depend on $x$ or $y$ — the plane looks the same everywhere, as shown in Figure 2. Putting this all together, we conclude that \begin{eqnarray*} \BB = B_y(z) \,\yhat \end{eqnarray*}
Recall Ampère's Law, which relates the circulation of the magnetic field around any closed loop to the current enclosed by the loop, that is, \begin{eqnarray*} \oint\BB \cdot d\rr = \mu_0 I \end{eqnarray*} We can use this to find $\BB$ by choosing an appropriate loop. Since $\BB$ points in the $y$-direction, choose a rectangular loop, two of whose sides are parallel to the $y$-axis. Since $B_y$ only depends on $z$, these two sides should be at different $z$ values. In other words, choose a loop in the $yz$-plane. Evaluate the LHS of Ampère's Law. The two sides parallel to the $z$-axis don't contribute, and each of the other two sides contributes $B_y(z)$ times the length of the side.
Assuming the loop does not intersect the $xy$-plane, the RHS vanishes; this forces $B_y$ to be constant. Well, not quite: this forces $B_y$ to be constant on each side of the $xy$-plane, but doesn't show that it's the same constant on both sides. In fact, there is another symmetry argument which says this can't be the case. Take the $xy$-plane, and rotate it $180^\circ$ about the $x$-axis. This doesn't change the current, but it takes, for instance, $\yhat$ above the plane to $-\yhat$ below.
So consider a loop which does intersect the $xy$-plane. Now the contributions on the two sides parallel to the $y$-axis will add. Orient the loop in the $-\yhat$ direction for $z>0$; this is the correct “right-hand rule” orientation for Ampère's Law. Let $B_y$ denote the (constant) magnitude of the magnetic field for $z>0$. Then the LHS of Ampère's Law yields $-2B_y$, times the length of the loop in the $y$ direction. But the RHS is the current flowing through the loop, which is \begin{eqnarray*} I = \int \KK\cdot\nn \, ds \end{eqnarray*} where the integral is taken along the intersection of the loop with the plane, and where $\nn=\xhat$. The current is therefore $|\KK|$ times the length, and we conclude that $-2B_y = |\KK|$, or equivalently \begin{eqnarray*} \BB = -\frac{\mu_0}{2} \,|\KK|\,\yhat \qquad (z>0) \end{eqnarray*} and of course $B_y$ has the opposite sign for $z<0$.