You are here: start » book » physcontent » vlinefinite
As an example of finding the potential due to a continuous charge source, let's calculate the potential the distance $r$ from the center of a uniform line segment of charge with total length $2L$. We will idealize the line segment as infinitely thin and describe it by the constant linear charge density $\lambda$. Because we are chopping a one-dimensional source into little lengths, $d\tau$ reduces to $|d\rr|$. \begin{equation} V(\rr)=\frac{1}{4\pi\epsilon_0}\int \frac{\lambda |d\rr|}{|\rr-\rrp|} \end{equation}
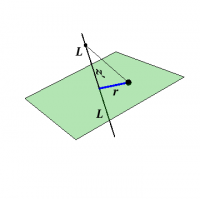
We will choose to work in cylindrical coordinates, centering the line segment on the $z$-axis and will find the potential at a distance $r$ from the origin in the $x$, $y$-plane, as shown in Figure 1. Now, we want to plug in information, using the “use what you know” strategy.
- The source lies along the $z$-axis at points with coordinates $r'=0$, $\phi'=0$, and $z'$.
- $|d\rr|$ becomes $dz'$ and the integral runs from $z'=-L$ to $z'=L$.
- For a voltmeter probe located on the $x$, $y$-plane, we have $z=0$.
- Because of the cylindrical symmetry, we can choose to evaluate the integral with the voltmeter probe at any $\phi$, so we will choose $\phi=0$ for simplicity. (It is an illuminating exercise to solve the integral for arbitrary $\phi$ and see how the algebra ends up reflecting the cylindrical symmetry.)
- In cylindrical coordinates (see homework problem: DistanceCurvilinear), the denominator is $\sqrt{r^2+r'^2-2r r' \cos(\phi-\phi')+(z-z')^2}$ which reduces to $\sqrt{r^2+z'^2}$. Alternatively, this result can be obtained directly from a diagram.