You are here: start » book » math » powerapproxhint
Wrap-Up: Using Technology to Visualize Power Series Approximations
See Figure 1 below. 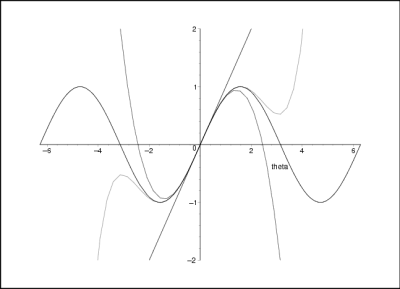 Figure 1: The function $\sin\theta$ and three approximations formed from
truncating the power series after the first, second, and third
non-zero terms (i.e. at the first, third, and fifth order.)
Figure 1: The function $\sin\theta$ and three approximations formed from
truncating the power series after the first, second, and third
non-zero terms (i.e. at the first, third, and fifth order.)
Notice that if you truncate a power series, then you are looking at a polynomial whose dominant term (i.e. highest power) is the highest term that you kept in the power series. Since $\sin\theta$ is bounded for large $\theta$ and a polynomial in $\theta$ is not bounded, the first few terms of the power series will be a terrible approximation for the function if $|\theta|$ is large enough. However, as you keep more and more terms in the series, the truncated series is a better and better approximation for the function for a larger and larger range of values of $\theta$.
A function $f(z)$ is called symmetric if the graph of the function for negative values of its argument is the mirror reflection (with the vertical axis acting as mirror) of the graph of the function for positive values of its argument, i.e. if $f(-z)=f(z)$. Similarly, a function is called anti-symmetric if the graph of the function for negative values of its argument is the negative of the mirror reflection (with the vertical axis acting as mirror) of the graph of the function for positive values of its argument, i.e. if $f(-z)=-f(z)$. All even positive integer powers of $z$ are symmetric; all odd positive integer powers are anti-symmetric. Since the function $f(\theta)=\sin\theta$ is an odd function of $\theta$, only the odd powers of $\theta$ appear in the power series expansion. You can see this antisymmetry in Figure 1 above.
This computer algebra worksheet began by approximating $\sin\theta$ by $\theta$ near the origin — which ought to be familiar. Higher order terms add more “bumps” to the curve; the graph of $\sin\theta$ has infinitely many bumps. It is remarkable that the power series for $\sin\theta$ converges for all values of $\theta$.
You can (and should) also make a power series approximation that fits a function well somewhere other than at the origin by writing the power series expanded “around” some other point such as $\theta=\frac{\pi}{6}$.